2022 College Scholastic Ability Test

Mathematics

Multiple Choice Questions
What is the value of \(\big(2^\sqrt{3}\times 4\big)^{\sqrt{3}-2}\)? [2 points]
  1. \(\dfrac{1}{4}\)
  2. \(\dfrac{1}{2}\)
  3. \(1\)
  4. \(2\)
  5. \(4\)
For the function \(f(x)=x^3+3x^2+x-1\), what is the value of \(f'(1)\)? [2 points]
  1. \(6\)
  2. \(7\)
  3. \(8\)
  4. \(9\)
  5. \(10\)
An arithmetic progression \(\{a_n\}\) satisfies
\(a_2=6\:\) and \(\:a_4+a_6=36\).
What is the value of \(a_{10}\)? [3 points]
  1. \(30\)
  2. \(32\)
  3. \(34\)
  4. \(36\)
  5. \(38\)
The graph of the function \(y=f(x)\) is as the figure below.
What is the value of \(\displaystyle\lim_{x\to-1-}\!f(x)+\lim_{x\to2}\!f(x)\)? [3 points]
  1. \(1\)
  2. \(2\)
  3. \(3\)
  4. \(4\)
  5. \(5\)

Mathematics

A sequence \(\{a_n\}\) with an initial term of \(1\) satisfies
\(a_{n+1}=\begin{cases} 2a_n &\; (a_n<7)\\\\ a_n-7 &\; (a_n\geq7) \end{cases}\)
for all positive integers \(n\). What is the value of \(\displaystyle\sum_{k=1}^8 a_k\)? [3 points]
  1. \(30\)
  2. \(32\)
  3. \(34\)
  4. \(36\)
  5. \(38\)
What is the number of integers \(k\) for which the equation \(2x^3-3x^2-12x+k=0\) has three distinct real solutions? [3 points]
  1. \(20\)
  2. \(23\)
  3. \(26\)
  4. \(29\)
  5. \(32\)
Suppose \(\pi<\theta<\dfrac{3}{2}\pi\). Given that \(\tan\!\theta-\dfrac{6}{\tan\!\theta}=1\), what is the value of \(\sin\theta+\cos\theta\)? [3 points]
  1. \(-\dfrac{2\sqrt{10}}{5}\)
  2. \(-\dfrac{\sqrt{10}}{5}\)
  3. \(0\)
  4. \(\dfrac{\sqrt{10}}{5}\)
  5. \(\dfrac{2\sqrt{10}}{5}\)

Mathematics

A line \(x=k\) bisects the region enclosed by the curve \(y=x^2-5x\) and the line \(y=x\). What is the value of the constant \(k\)? [3 points]
  1. \(3\)
  2. \(\dfrac{13}{4}\)
  3. \(\dfrac{7}{2}\)
  4. \(\dfrac{15}{4}\)
  5. \(4\)
Let \(\mathrm{P}\) and \(\mathrm{Q}\) be points where the line \(y=2x+k\) meets the two functions
\(y=\left(\!\dfrac{2}{3}\!\right)^{\!x+3}\!\!+1\:\) and \(\:y=\left(\!\dfrac{2}{3}\!\right)^{\!x+1}\!\!+\dfrac{8}{3}\)
respectively. Given that \(\overline{\mathrm{PQ}}=\sqrt{5}\), what is the value of the constant \(k\)? [4 points]
  1. \(\dfrac{31}{6}\)
  2. \(\dfrac{16}{3}\)
  3. \(\dfrac{11}{2}\)
  4. \(\dfrac{17}{3}\)
  5. \(\dfrac{35}{6}\)
For a cubic function \(f(x)\), the tangent line to the curve \(y=f(x)\) at point \((0,0)\) on the curve is equal to the tangent line to the curve \(y=xf(x)\) at point \((1,2)\) on the curve. What is the value of \(f'(2)\)? [4 points]
  1. \(-18\)
  2. \(-17\)
  3. \(-16\)
  4. \(-15\)
  5. \(-14\)

Mathematics

For a positive number \(a\), a function \(f(x)\) is defined on the set \(\left\{x\middle|-\dfrac{a}{2}<x\leq a, x\ne\dfrac{a}{2}\right\}\) as
\(f(x)=\tan\dfrac{\pi x}{a}\).
As the figure shows, there is a line that passes through three points \(\mathrm{O, A}\) and \(\mathrm{B}\) on the graph of the function \(y=f(x)\). Let \(\mathrm{C}\) be the point where a line, passing through point \(\mathrm{A}\) parallel to the \(x\)-axis, meets the graph of the function \(y=f(x)\) again \((\mathrm{C}\ne \mathrm{A})\). Given that triangle \(\mathrm{ABC}\) is an equilateral triangle, what is the area of the triangle \(\mathrm{ABC}\)?
(※ \(\mathrm{O}\) is the origin.) [4 points]
  1. \(\dfrac{3\sqrt{3}}{2}\)
  2. \(\dfrac{17\sqrt{3}}{12}\)
  3. \(\dfrac{4\sqrt{3}}{3}\)
  4. \(\dfrac{5\sqrt{3}}{4}\)
  5. \(\dfrac{7\sqrt{3}}{6}\)
A function \(f(x)\) continuous on the set of all real numbers satisfies
\(\{f(x)\}^3-\{f(x)\}^2-x^2f(x)+x^2=0\)
for all real numbers \(x\). If the function \(f(x)\) has a global maximum value of \(1\) and a global minimum value of \(0\), what is the value of \(f\!\left(\!-\dfrac{4}{3}\!\right)+f(0)+f\!\left(\!\dfrac{1}{2}\!\right)\)? [4 points]
  1. \(\dfrac{1}{2}\)
  2. \(1\)
  3. \(\dfrac{3}{2}\)
  4. \(2\)
  5. \(\dfrac{5}{2}\)

Mathematics

On the \(xy\)-plane, for two constants \(a\) and \(b\) \((1<a<b)\), the \(y\)-intercept of a line that passes through two points \((a,\log_2 a)\) and \((b,\log_2 b)\) is equal to the \(y\)-intercept of a line that passes through two points \((a,\log_4 a)\) and \((b,\log_4 b)\). For the function \(f(x)=a^{bx}+b^{ax}\), if \(f(1)=40\), what is the value of \(f(2)\)? [4 points]
  1. \(760\)
  2. \(800\)
  3. \(840\)
  4. \(880\)
  5. \(920\)
Suppose a point \(\mathrm{P}\) is moving on the number line, and its position \(x(t)\) at time \(t\) is given as
\(x(t)=t(t-1)(at+b)\)
for two constants \(a\) and \(b\). If \(v(t)\), the velocity of point \(\mathrm{P}\) at time \(t\), satisfies \(\displaystyle\int_0^1|v(t)|dt=2\), which option only contains every correct statement in the <List>? [4 points]
  1. \(\displaystyle\int_0^1v(t)dt=0\)
  2. There exists a \(t_1\) in the open interval \((0,1)\) such that \(|x(t_1)|>1\).
  3. If \(|x(t)|<1\) for all \(t\) in \(0\leq t\leq 1\), then there exists a \(t_2\) in the open interval \((0,1)\) such that \(x(t_2)=0\).
  1. a
  2. a, b
  3. a, c
  4. b, c
  5. a, b, c

Mathematics

There are two circles \(C_1\) and \(C_2\) with radius \(\overline{\mathrm{O_1O_2}}\) whose centers are points \(\mathrm{O_1}\) and \(\mathrm{O_2}\) respectively. As the figure shows, there are three distinct points \(\mathrm{A, B}\) and \(\mathrm{C}\) on the circle \(C_1\), and a point \(\mathrm{D}\) on the circle \(\mathrm{C_2}\). Points \(\mathrm{A, O_1}\) and \(\mathrm{O_2}\) are on a line, and points \(\mathrm{C, O_2}\) and \(\mathrm{D}\) are on a line.
Let \(\angle\mathrm{BO_1A}\!=\!\theta_1, \angle\mathrm{O_2O_1C}\!=\!\theta_2\) and \(\angle\mathrm{O_1O_2D}\!=\!\theta_3\).
The following is a process computing the ratio of line segments \(\mathrm{AB}\) and \(\mathrm{CD}\), given that \(\overline{\mathrm{AB}}:\overline{\mathrm{O_1D}}=1:2\sqrt{2}\:\) and \(\:\theta_3=\theta_1+\theta_2\).
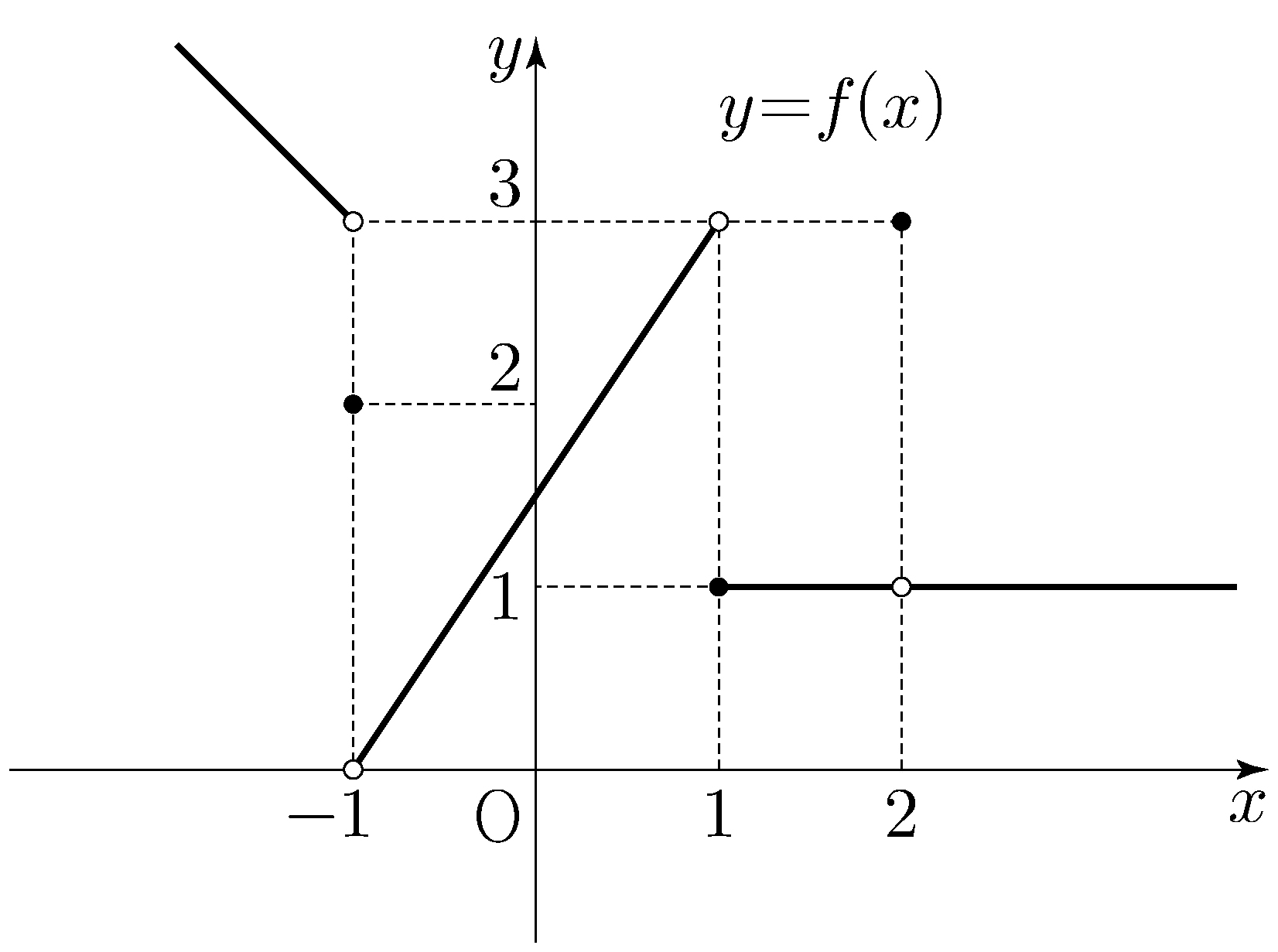
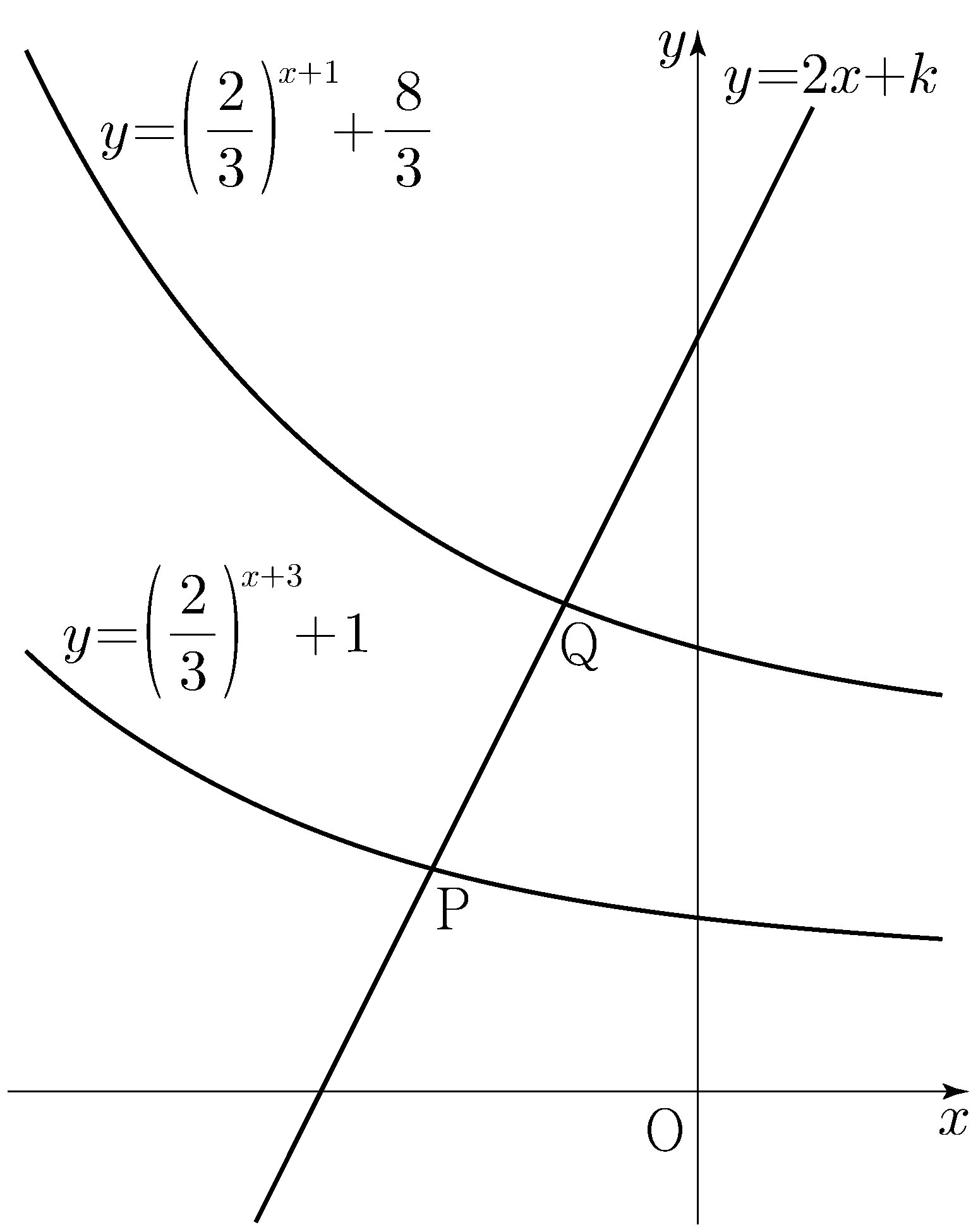
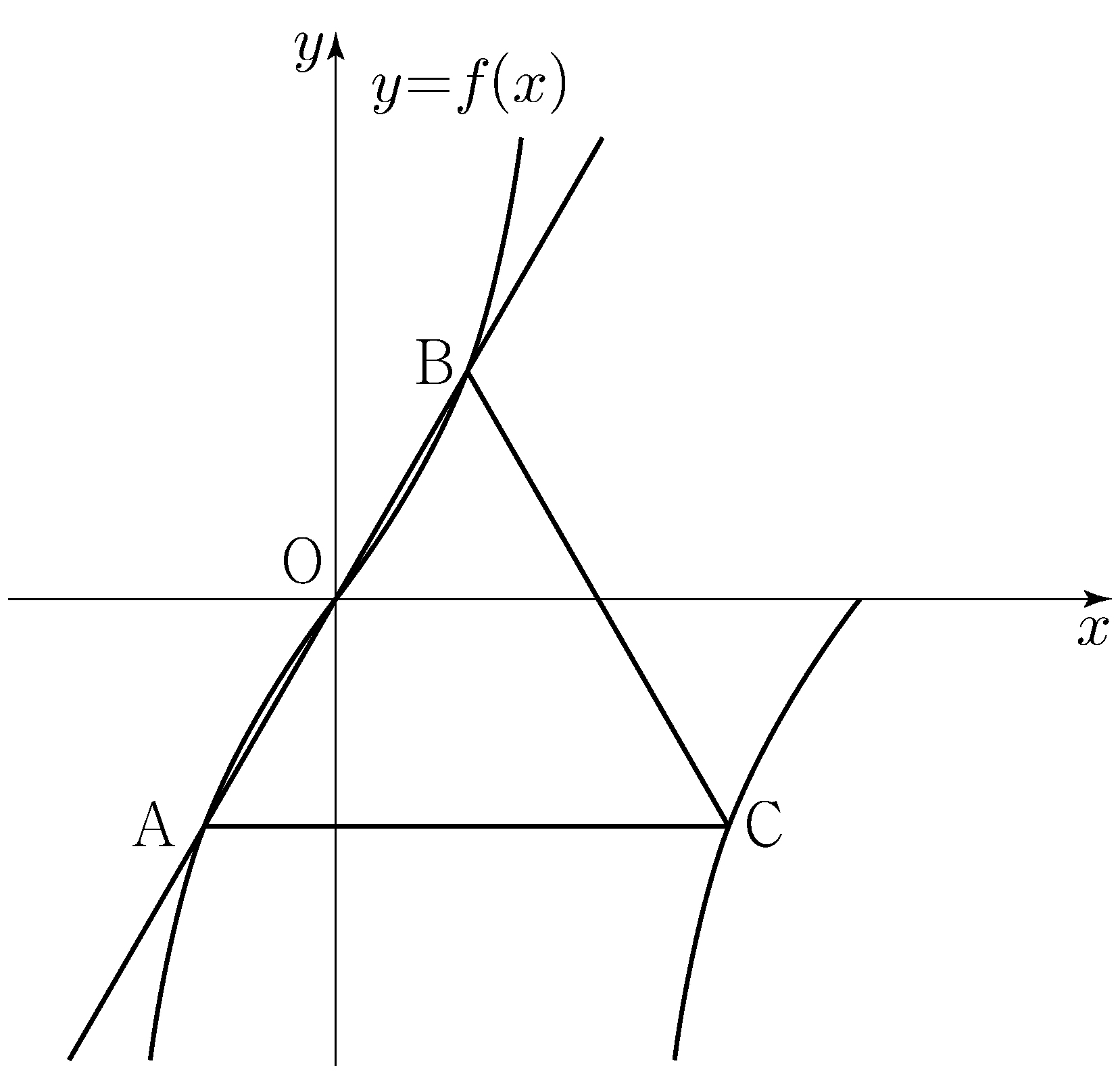
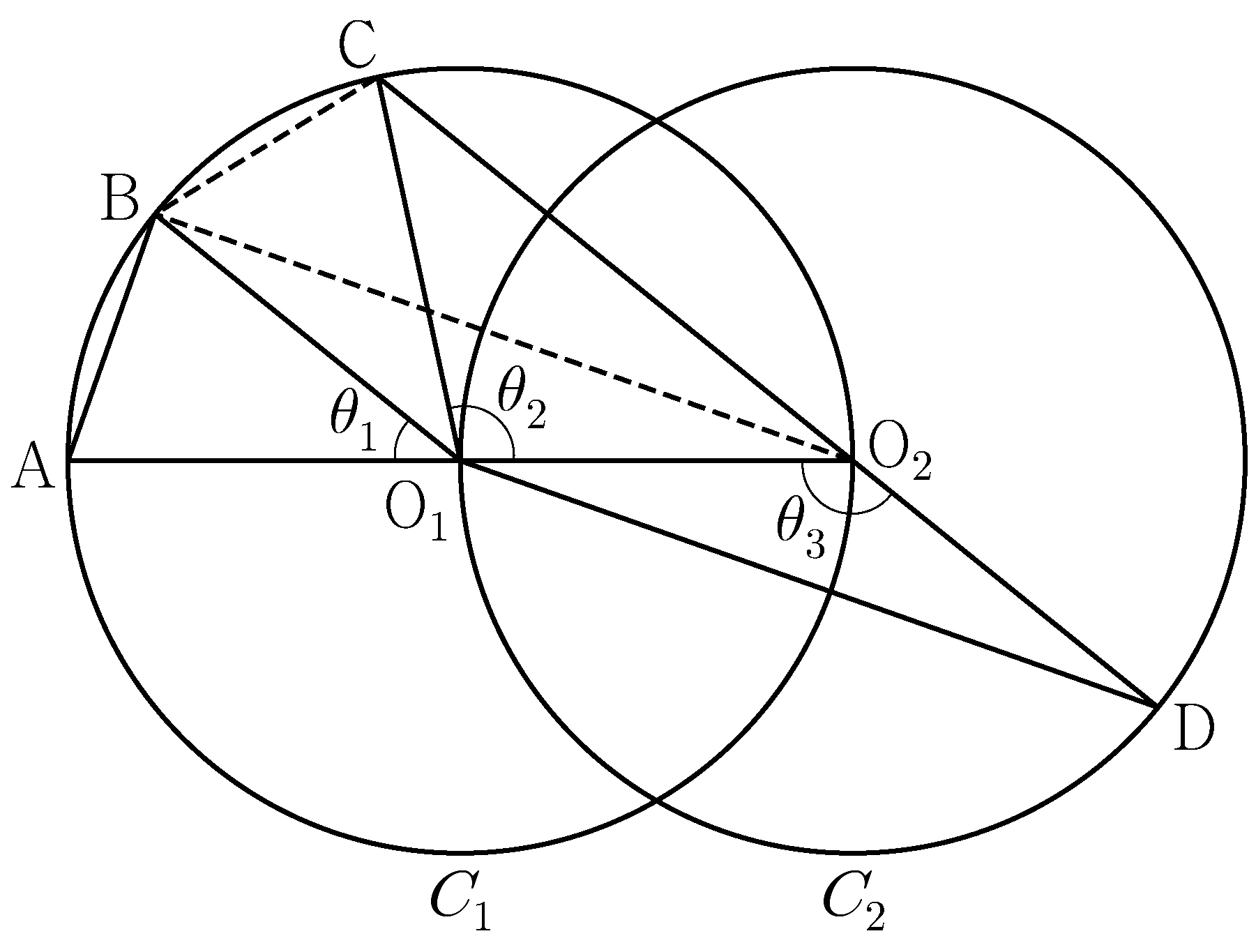
\(\angle\mathrm{CO_2O_1}+\angle\mathrm{O_1O_2D}=\pi\), so \(\theta_3=\dfrac{\pi}{2}+\dfrac{\theta_2}{2}\).
\(\theta_3=\theta_1+\theta_2\), so \(2\theta_1+\theta_2\!=\!\pi\) and \(\angle\mathrm{CO_1B}=\theta_1\).
Since \(\angle\mathrm{O_2O_1B}=\theta_1+\theta_2=\theta_3\),
triangles \(\mathrm{O_1O_2B}\) and \(\mathrm{O_1O_2B}\) are congruent. Suppose \(\overline{\mathrm{AB}}=k\).
Then \(\overline{\mathrm{BO_2}}=\overline{\mathrm{O_1D}}=2\sqrt{2}k\), so \(\overline{\mathrm{AO_2}}=\fbox{\(\;(\alpha)\;\)}\).
\(\angle\mathrm{BO_2A}=\dfrac{\theta_1}{2}\), therefore \(\cos\!\dfrac{\theta_1}{2}=\fbox{\(\;(\beta)\;\)}\).
In the triangle \(\mathrm{O_2BC}\),
\(\overline{\mathrm{BC}}=k, \overline{\mathrm{BO_2}}=2\sqrt{2}k\), and \(\angle\mathrm{CO_2B}=\dfrac{\theta_1}{2}\),
so \(\overline{\mathrm{O_2C}}=\fbox{\(\;(\gamma)\;\)}\) by the law of cosines.
\(\overline{\mathrm{CD}}=\overline{\mathrm{O_2D}}+\overline{\mathrm{O_2C}} =\overline{\mathrm{O_1O_2}}+\overline{\mathrm{O_2C}}\), therefore
\(\overline{\mathrm{AB}}:\overline{\mathrm{CD}}= k:\Big(\dfrac{\fbox{\(\;(\alpha)\;\)}}{2}+\fbox{\(\;(\gamma)\;\)}\,\Big)\).
Let \(f(t)\) and \(g(t)\) be the correct expression for \((\alpha)\) and \((\gamma)\) respectively, and let \(p\) be the correct number for \((\beta)\). What is the value of \(f(p)\times g(p)\)? [4 points]
  1. \(\dfrac{169}{27}\)
  2. \(\dfrac{56}{9}\)
  3. \(\dfrac{167}{27}\)
  4. \(\dfrac{166}{27}\)
  5. \(\dfrac{55}{9}\)
Short Answer Questions
Compute \(\log_2 120-\dfrac{1}{\log_{15}2}\). [3 points]
A function \(f(x)\) satisfies \(f'(x)=3x^2+2x\) and \(f(0)=2\). Compute \(f(1)\). [3 points]

Mathematics

A sequence \(\{a_n\}\) satisfies
\(\displaystyle\sum_{k=1}^{10}a_k - \sum_{k=1}^7 \dfrac{a_k}{2} = 56\)
and \(\:\displaystyle\sum_{k=1}^{10}2a_k - \sum_{k=1}^8 a_k = 100\).
Compute \(a_8\). [3 points]
The function \(f(x)=x^3+ax^2-(a^2-8a)x+3\) increases on the set of all real numbers. Compute the maximum value of the real number \(a\). [3 points]
A function \(f(x)\) differentiable on the set of all real numbers satisfies the following.
  1. \(f(x)=x\) on the closed interval \([0,1]\).
  2. For some constants \(a\) and \(b\), \(f(x+1)-xf(x)=ax+b\) on the interval \([0,\infty)\).
Compute \(\displaystyle 60\times\int_1^2 f(x)dx\). [4 points]

Mathematics

A sequence \(\{a_n\}\) satisfies the following.
  1. \(\big|a_1\big|=2\)
  2. \(\big|a_{n+1}\big| = 2\big|a_n\big|\) for all positive integers \(n\).
  3. \(\displaystyle\sum_{n=1}^{10}a_n=-14\)
Compute \(a_1+a_3+a_5+a_7+a_9\). [4 points]
For a cubic function \(f(x)\) with a leading coefficient of \(\dfrac{1}{2}\) and for a real number \(t\), let \(g(t)\) be the number of real solutions to the equation \(f'(x)=0\) in the closed interval \([t,t+2]\). \(g(x)\) satisfies the following.
  1. \(\displaystyle\lim_{t\to a+}\!g(t)\!+\!\lim_{t\to a-}\!g(t)\leq 2\) for all real numbers \(a\).
  2. \(g(f(1))=g(f(4))=2\:\) and \(\:g(f(0))=1\).
Compute \(f(5)\). [4 points]

2022 College Scholastic Ability Test

Mathematics (Prob. & Stat.)

Multiple Choice Questions
In the expansion of the polynomial \((x+2)^7\), what is the coefficient of \(x^5\)? [2 points]
  1. \(42\)
  2. \(56\)
  3. \(70\)
  4. \(84\)
  5. \(98\)
A random variable \(X\) follows the binomial distribution \(\mathrm{B}\!\left(\!n,\dfrac{1}{3}\!\right)\). If \(\mathrm{V}(2X)=40\), what is the value of \(n\)? [3 points]
  1. \(30\)
  2. \(35\)
  3. \(40\)
  4. \(45\)
  5. \(50\)

Mathematics (Prob. & Stat.)

What is the number of \(5\)-tuples \((a,b,c,d,e)\) where \(a,b,c,d\) and \(e\) are positive integers that satisfy the following? [3 points]
  1. \(a+b+c+d+e=12\)
  2. \(\big|a^2-b^2\big|=5\)
  1. \(30\)
  2. \(32\)
  3. \(34\)
  4. \(36\)
  5. \(38\)
There is a sack containing \(10\) cards marked with integers from \(1\) to \(10\) respectively. Suppose we randomly take out \(3\) cards from this sack at the same time. What is the probability that the smallest number among the three integers on the cards taken out is less than \(5\) or greater than \(6\)? [3 points]
  1. \(\dfrac{4}{5}\)
  2. \(\dfrac{5}{6}\)
  3. \(\dfrac{13}{15}\)
  4. \(\dfrac{9}{10}\)
  5. \(\dfrac{14}{15}\)

Mathematics (Prob. & Stat.)

The \(1\)-charge mileage of electric cars produced in some company follows the normal distribution with a mean of \(m\) and a standard deviation of \(\sigma\).
Suppose \(100\) electric cars produced in this company were randomly sampled, and the sample mean of the
\(1\)-charge mileage was \(\overline{x_1}\). A \(95\%\) confidence interval for \(m\) computed with this sample is \(a\leq m\leq b\).
Suppose \(400\) electric cars produced in this company were randomly sampled, and the sample mean of the
\(1\)-charge mileage was \(\overline{x_2}\). A \(99\%\) confidence interval for \(m\) computed with this sample is \(c\leq m\leq d\).
Given that \(\overline{x_1}-\overline{x_2}=1.34\) and \(a=c\), what is the value of \(b-a\)? (※ The unit of mileage is \(\text{km}\), and for a random variable \(Z\) that follows the standard normal distribution, suppose \(\mathrm{P}(|Z| \leq 1.96) = 0.95\) and \(\mathrm{P}(|Z| \leq 2.58) = 0.99\).) [3 points]
  1. \(5.88\)
  2. \(7.84\)
  3. \(9.80\)
  4. \(11.76\)
  5. \(13.72\)
For sets \(X=\{1,2,3,4,5\}\) and \(Y=\{1,2,3,4\}\), what is the number of functions \(f\) from \(X\) to \(Y\) that satisfy the following? [4 points]
  1. \(f(x)\geq \sqrt{x}\) for all elements \(x\) in set \(X\).
  2. The image of the function \(f\) has \(3\) elements.
  1. \(128\)
  2. \(138\)
  3. \(148\)
  4. \(158\)
  5. \(168\)

Mathematics (Prob. & Stat.)

Short Answer Questions
Two absolutely continuous random variables \(X\) and \(Y\) take the value of \(0\leq X\leq6\) and \(0\leq Y\leq6\). The probability density function of \(X\) and \(Y\) are \(f(x)\) and \(g(x)\) respectively. The graph of \(f(x)\) is as the figure below.
For all \(x\) in \(0\leq x\leq 6\),
\(f(x)+g(x)=k\) (\(k\) is a constant)
is satisfied. Given that \(\mathrm{P}(6k\leq Y\leq 15k)=\dfrac{q}{p}\), Compute \(p+q\). (※ \(p\) and \(q\) are positive integers that are coprime.) [4 points]
There is an empty sack and a basket containing at least \(10\) white balls and black balls each. Let us perform the following trial with a die.
Throw the die once.
If the number it lands on is \(5\) or more,
move \(2\) white balls from the basket to the sack.
If the number it lands on is \(4\) or less,
move \(1\) black ball from the basket to the sack.
Let us repeat the trial above \(5\) times. Let \(a_n\) and \(b_n\) be the number of white balls and black balls in the sack, respectively, after the \(n\)th trial \((1\leq n\leq5)\).
Given that \(a_5+b_5\geq 7\), the probability that there exists an integer \(k\) \((1\leq k\leq5)\) such that \(a_k=b_k\),
is equal to \(\dfrac{q}{p}\). Compute \(p+q\). (※ \(p\) and \(q\) are positive integers that are coprime.) [4 points]

2022 College Scholastic Ability Test

Mathematics (Calculus)

Multiple Choice Questions
What is the value of \( \displaystyle\lim_{n\to\infty} \dfrac{ \dfrac{5}{n}+\dfrac{3}{n^2} }{ \dfrac{1}{n}-\dfrac{2}{n^3} } \)? [2 points]
  1. \(1\)
  2. \(2\)
  3. \(3\)
  4. \(4\)
  5. \(5\)
A function \(f(x)\) differentiable on the set of all real numbers satisfies
\(f\big(x^3+x\big)=e^x\)
for all real numbers \(x\). What is the value of \(f'(2)\)? [3 points]
  1. \(e\)
  2. \(\dfrac{e}{2}\)
  3. \(\dfrac{e}{3}\)
  4. \(\dfrac{e}{4}\)
  5. \(\dfrac{e}{5}\)

Mathematics (Calculus)

A geometric progression \(\{a_n\}\) satisfies
\(\displaystyle\sum_{n=1}^\infty (a_{2n-1}-a_{2n})=3\:\) and \(\:\displaystyle\sum_{n=1}^\infty {a_n}^2=6\).
What is the value of \(\displaystyle\sum_{n=1}^\infty a_n\)? [3 points]
  1. \(1\)
  2. \(2\)
  3. \(3\)
  4. \(4\)
  5. \(5\)
What is the value of \( \displaystyle\lim_{n\to\infty}\sum_{k=1}^n \dfrac{k^2+2kn}{k^3+3k^2n+n^3} \)? [3 points]
  1. \(\ln5\)
  2. \(\dfrac{\ln5}{2}\)
  3. \(\dfrac{\ln5}{3}\)
  4. \(\dfrac{\ln5}{4}\)
  5. \(\dfrac{\ln5}{5}\)

Mathematics (Calculus)

Suppose a point \(\mathrm{P}\) is moving on the \(xy\)-plane, and its position at time \(t \, (t > 0)\) is the midpoint of two intersections of the curve \(y=x^2\) and the line \(y=t^2x-\dfrac{\ln t}{8}\). What is the distance that point \(\mathrm{P}\) travels from time \(t=1\) to \(t=e\)? [3 points]
  1. \(\dfrac{e^4}{2}-\dfrac{3}{8}\)
  2. \(\dfrac{e^4}{2}-\dfrac{5}{16}\)
  3. \(\dfrac{e^4}{2}-\dfrac{1}{4}\)
  4. \(\dfrac{e^4}{2}-\dfrac{3}{16}\)
  5. \(\dfrac{e^4}{2}-\dfrac{1}{8}\)
For the function \(f(x)=6\pi(x-1)^2\), define the function \(g(x)\) as
\(g(x)=3f(x)+4\cos f(x)\).
What is the number of values \(x\) in \(0<x<2\) where the function \(g(x)\) has a local minimum? [4 points]
  1. \(6\)
  2. \(7\)
  3. \(8\)
  4. \(9\)
  5. \(10\)

Mathematics (Calculus)

Short Answer Questions
As the figure shows, there is a semicircle whose diameter is the line segment \(\mathrm{AB}\) with a length of \(2\). Let \(\mathrm{P}\) and \(\mathrm{Q}\) be points on arc \(\mathrm{AB}\) such that \(\angle\mathrm{PAB}=\theta\) and \(\angle\mathrm{QBA}=2\theta\). Let \(\mathrm{R}\) be the intersection of two line segments \(\mathrm{AP}\) and \(\mathrm{BQ}\). Let \(\mathrm{S, T}\) and \(\mathrm{U}\) be points on line segments \(\mathrm{AB, BR}\) and \(\mathrm{AR}\) respectively, such that lines \(\mathrm{UT}\) and \(\mathrm{AB}\) are parallel and the triangle \(\mathrm{STU}\) is an equilateral triangle. Let \(f(\theta)\) be the area of the region enclosed by the arc \(\mathrm{AQ}\) and two line segments \(\mathrm{AR}\) and \(\mathrm{QR}\). Let \(g(\theta)\) be the area of the triangle \(\mathrm{STU}\).
Given that \(\displaystyle\lim_{\theta\to 0+}\!\dfrac{g(\theta)}{\theta\times\!f(\theta)}=\dfrac{q}{p}\sqrt{3}\), compute \(p+q\).

(※ \(0<\theta<\dfrac{\pi}{6}\), and \(p\) and \(q\) are positive integers that are coprime.) [4 points]
A strictly increasing function \(f(x)\) differentiable on the set of all real numbers, satisfies the following.
  1. \(f(1)=1\:\) and \(\:\displaystyle\int_1^2f(x)dx=\dfrac{5}{4}\).
  2. Let \(g(x)\) be the inverse of the function \(f(x)\). Then \(g(2x)=2f(x)\) for all \(x\geq1\).
Given that \(\displaystyle\int_1^8xf'(x)dx=\dfrac{q}{p}\), compute \(p+q\).
(※ \(p\) and \(q\) are positive integers that are coprime.) [4 points]

2022 College Scholastic Ability Test

Mathematics (Geometry)

Multiple Choice Questions
In \(3\)-dimensional space, let point \(\mathrm{P}\) be the reflection of point \(\mathrm{A}(2,1,3)\) about the \(xy\)-plane, and let point \(\mathrm{Q}\) be the reflection of point \(\mathrm{A}\) about the
\(yz\)-plane. What is the length of the line segment \(\mathrm{PQ}\)? [2 points]
  1. \(5\sqrt{2}\)
  2. \(2\sqrt{13}\)
  3. \(3\sqrt{6}\)
  4. \(2\sqrt{14}\)
  5. \(2\sqrt{15}\)
What is the length of the major axis of the hyperbola \(\dfrac{x^2}{a^2}-\dfrac{y^2}{6}=1\), if one of its foci has coordinates \((3\sqrt{2}, 0)\)? (※ \(a\) is a positive number.) [3 points]
  1. \(3\sqrt{3}\)
  2. \(\dfrac{7\sqrt{3}}{2}\)
  3. \(4\sqrt{3}\)
  4. \(\dfrac{9\sqrt{3}}{2}\)
  5. \(5\sqrt{3}\)

Mathematics (Geometry)

On the \(xy\)-plane, let \(\theta\) be the acute angle between two lines
\(\dfrac{x+1}{2}=y-3\:\) and \(\:x-2=\dfrac{y-5}{3}\).
What is the value of \(\cos\theta\)? [3 points]
  1. \(\dfrac{1}{2}\)
  2. \(\dfrac{\sqrt{5}}{4}\)
  3. \(\dfrac{\sqrt{6}}{4}\)
  4. \(\dfrac{\sqrt{7}}{4}\)
  5. \(\dfrac{\sqrt{2}}{2}\)
Consider the ellipse \(\dfrac{x^2}{64}+\dfrac{y^2}{16}=1\) with two foci \(\mathrm{F}\) and \(\mathrm{F'}\), and a point \(\mathrm{A}\) on the ellipse in the \(1\)st quadrant. Let \(C\) be a circle tangent to two lines \(\mathrm{AF}\) and \(\mathrm{AF'}\) at the same time, which has a center on the \(y\)-axis with a negative \(y\)-coordinate. Let \(\mathrm{B}\) be the center of the circle \(C\). Given that the quadrilateral \(\mathrm{AFBF'}\) has an area of \(72\), what is the radius of the circle \(C\,\)? [3 points]
  1. \(\dfrac{17}{2}\)
  2. \(9\)
  3. \(\dfrac{19}{2}\)
  4. \(10\)
  5. \(\dfrac{21}{2}\)

Mathematics (Geometry)

As the figure shows, there is a cube \(\mathrm{ABCD-EFGH}\) with side lengths of \(4\). Let \(\mathrm{M}\) be the midpoint of the line segment \(\mathrm{AD}\). What is the area of the triangle \(\mathrm{MEG}\)? [3 points]
  1. \(\dfrac{21}{2}\)
  2. \(11\)
  3. \(\dfrac{23}{2}\)
  4. \(12\)
  5. \(\dfrac{25}{2}\)
For two positive numbers \(a\) and \(p\), let \(\mathrm{F_1}\) be the focus of the parabola \((y-a)^2=4px\), and let \(\mathrm{F_2}\) be the focus of the parabola \(y^2=-4x\). Let \(\mathrm{P}\) and \(\mathrm{Q}\) be points where the line segment \(\mathrm{F_1F_2}\) meets the two parabola respectively. Given that \(\overline{\mathrm{F_1F_2}}=3\) and \(\overline{\mathrm{PQ}}=1\), what is the value of \(a^2+p^2\)? [4 points]
  1. \(6\)
  2. \(\dfrac{25}{4}\)
  3. \(\dfrac{13}{2}\)
  4. \(\dfrac{27}{4}\)
  5. \(7\)

Mathematics (Geometry)

Short Answer Questions
On the \(xy\)-plane, there is a parallelogram \(\mathrm{ABCD}\) where \(\overline{\mathrm{OA}}=\sqrt{2}, \overline{\mathrm{OB}}=2\sqrt{2}\) and \(\cos(\angle\mathrm{AOB})=\dfrac{1}{4}\).
A point \(\mathrm{P}\) satisfies the following.
  1. \(\overrightarrow{\mathrm{OP}}= s\,\overrightarrow{\mathrm{OA}}+t\,\overrightarrow{\mathrm{OB}} \; (0\leq s\leq1, 0\leq t\leq1)\)
  2. \(\overrightarrow{\mathrm{OP}}\cdot\overrightarrow{\mathrm{OB}}+ \overrightarrow{\mathrm{BP}}\cdot\overrightarrow{\mathrm{BC}}=2\)
Consider a circle with center \(\mathrm{O}\) that passes through point \(\mathrm{A}\). For a point \(\mathrm{X}\) moving on this circle, Let \(M\) and \(m\) be the maximum value and minimum value of \(\big|3\overrightarrow{\mathrm{OP}}-\overrightarrow{\mathrm{OX}}\big|\) respectively.
Given that \(M\times m=a\sqrt{6}+b\), compute \(a^2+b^2\).
(※ \(a\) and \(b\) are rational numbers.) [4 points]
In \(3\)-dimensional space, consider a sphere
\(S:\,(x-2)^2+(y-\sqrt{5})^2+(z-5)^2=25\)
with center \(\mathrm{C}(2,\sqrt{5},5)\) that passes through point \(\mathrm{P}(0,0,1)\). Suppose a point \(\mathrm{Q}\) is moving on a circle which is the intersection of sphere \(S\) and plane \(\mathrm{OPC}\), and a point \(\mathrm{R}\) is moving on the sphere \(S\). Let \(\mathrm{Q_1}\) and \(\mathrm{R_1}\) be the projections of points \(\mathrm{Q}\) and \(\mathrm{R}\) onto the
\(xy\)-plane respectively.
For the points \(\mathrm{Q}\) and \(\mathrm{R}\) that maximizes the area of the triangle \(\mathrm{OQ_1R_1}\), the projection of triangle \(\mathrm{OQ_1R_1}\) onto plane \(\mathrm{PQR}\) has an area of \(\dfrac{q}{p}\sqrt{6}\). Compute \(p+q\).
(※ \(\mathrm{O}\) is the origin. Three points \(\mathrm{O, Q_1}\) and \(\mathrm{R_1}\) are not on a line. \(p\) and \(q\) are positive integers that are coprime.) [4 points]