Consider the \(60\) balls in the sack as a population.
Let the random variable \(X\) be the number
marked on a ball randomly taken out from this population.
The distribution of \(X\), which is the distribution of the population,
is as the table below.
| \(X\) |
\(1\) |
\(2\) |
\(3\) |
Total |
| \(\mathrm{P}(X=x)\) |
\(\dfrac{1}{6}\) |
\(\dfrac{1}{3}\) |
\(\dfrac{1}{2}\) |
\(1\) |
Therefore the mean \(m\) and variance \(\sigma^2\)
of the population are
\(m=\mathrm{E}(X)=\dfrac{7}{3}\:\) and
\(\:\sigma^2=\mathrm{V}(X)=\fbox{\(\;(\alpha)\;\)}\).
Let \(\overline{X}\) be the mean of a random sample of size \(10\)
from this population. Then,
\(\mathrm{E}(\overline{X})=\dfrac{7}{3}\:\) and
\(\:\mathrm{V}(\overline{X})=\fbox{\(\;(\beta)\;\)}\).
Let \(X_n\) be the number marked on the \(n\)th ball
taken out from the sack. Then,
\(\displaystyle Y=\sum_{n=1}^{10}X_n=10\overline{X}\)
therefore
\(\mathrm{E}(Y)=\dfrac{70}{3}\:\) and
\(\:\mathrm{V}(Y)=\fbox{\(\;(\gamma)\;\)}\).
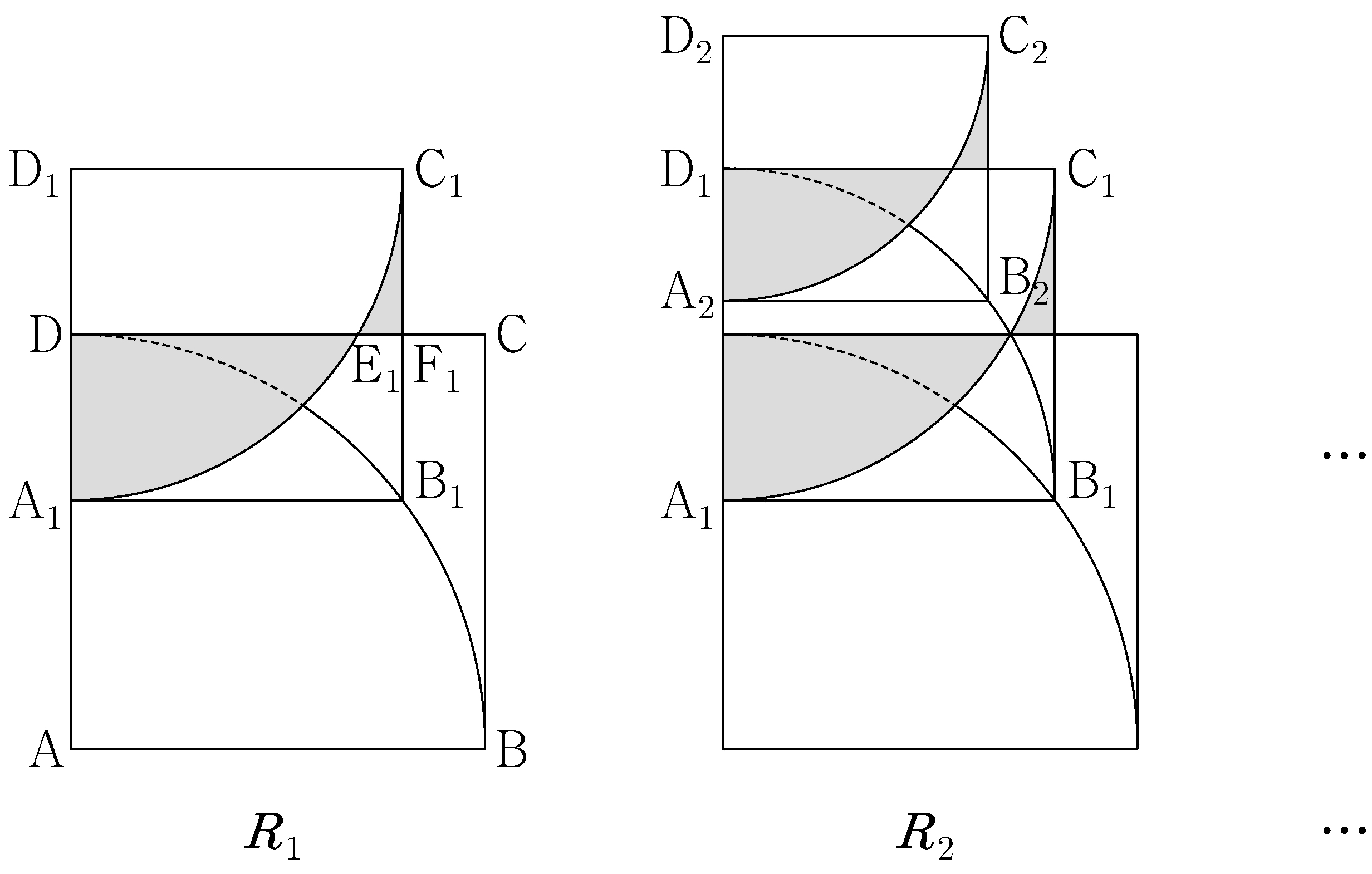
 shape consisting of
the region enclosed by arc \(\mathrm{A_1E_1}\) and lines \(\mathrm{DA_1}\) and \(\mathrm{DE_1}\),
and the region enclosed by arc \(\mathrm{E_1C_1}\) and lines \(\mathrm{E_1F_1}\) and \(\mathrm{F_1C_1}\).
shape consisting of
the region enclosed by arc \(\mathrm{A_1E_1}\) and lines \(\mathrm{DA_1}\) and \(\mathrm{DE_1}\),
and the region enclosed by arc \(\mathrm{E_1C_1}\) and lines \(\mathrm{E_1F_1}\) and \(\mathrm{F_1C_1}\).