2019 College Scholastic Ability Test

Mathematics (Type Ga)

Multiple Choice Questions
For two vectors \(\vec{a} = (1,-2)\) and \(\vec{b} = (-1, 4)\), what is the sum of all components of the vector \(\vec{a} + 2\vec{b}\)? [2 points]
  1. \(1\)
  2. \(2\)
  3. \(3\)
  4. \(4\)
  5. \(5\)
What is the value of \(\displaystyle\lim_{x\to0} \dfrac{x^2+5x}{\ln (1+3x)}\)? [2 points]
  1. \(\dfrac{7}{3}\)
  2. \(2\)
  3. \(\dfrac{5}{3}\)
  4. \(\dfrac{4}{3}\)
  5. \(1\)
For two points \(\mathrm{A}(2, a, -2)\) and \(\mathrm{B}(5, 2, -1)\) in
\(3\)-dimensional space, if the point internally dividing the line segment \(\mathrm{AB}\) in the ratio \(2:1\) is on the
\(x\)-axis, what is the value of \(a\)? [2 points]
  1. \(1\)
  2. \(2\)
  3. \(3\)
  4. \(4\)
  5. \(5\)
Consider two events \(A\) and \(B\) where \(A\) and \(B\,^C\) are mutually exclusive. Given that
\(\mathrm{P}(A) = \dfrac{1}{3}\, \) and \(\, \mathrm{P}(A^C \cap B) = \dfrac{1}{6}\),
what is the value of \(\mathrm{P}(B)\)?
(※ \(A^C\) is the complement of \(A\).) [3 points]
  1. \(\dfrac{5}{12}\)
  2. \(\dfrac{1}{2}\)
  3. \(\dfrac{7}{12}\)
  4. \(\dfrac{2}{3}\)
  5. \(\dfrac{3}{4}\)

Mathematics (Type Ga)

The graph of the function \(y=2^x + 2\) translated
\(m\) units horizontally, and the graph of the function \(y=\log_2 8x\) translated \(2\) units horizontally,
are reflections about the line \(y=x\).
What is the value of the constant \(m\)? [3 points]
  1. \(1\)
  2. \(2\)
  3. \(3\)
  4. \(4\)
  5. \(5\)
If a point \(\mathrm{P}\) on the parabola \(y^2=12x\) with focus \(\mathrm{F}\) satisfies \(\overline{\mathrm{PF}}=9\), what is the \(x\)-coordinate of point \(\mathrm{P}\)? [3 points]
  1. \(6\)
  2. \(\dfrac{13}{2}\)
  3. \(7\)
  4. \(\dfrac{15}{2}\)
  5. \(8\)
What is the slope of the tangent line to the curve \(e^x - xe^y = y\) at point \((0, 1)\)? [3 points]
  1. \(3-e\)
  2. \(2-e\)
  3. \(1-e\)
  4. \(-e\)
  5. \(-1-e\)

Mathematics (Type Ga)

If a random variable \(X\) following the binomial distribution \(\mathrm{B}\!\left(\!n, \dfrac{1}{2}\!\right)\) satisfies \(\mathrm{E}(X^2) = \mathrm{V}(X) + 25\), what is the value of \(n\)? [3 points]
  1. \(10\)
  2. \(12\)
  3. \(14\)
  4. \(16\)
  5. \(18\)
Let \(g(x)\) be the inverse function of the function \(f(x) = \dfrac{1}{1+e^{-x}}\). What is the value of \(g'(f(-1))\)? [3 points]
  1. \(\dfrac{1}{(1+e)^2}\)
  2. \(\dfrac{e}{1+e}\)
  3. \(\left(\dfrac{1+e}{e}\right)^2\)
  4. \(\dfrac{e^2}{1+e}\)
  5. \(\dfrac{(1+e)^2}{e}\)
There is a sack containing \(7\) marbles marked with integers from \(2\) to \(8\) respectively.
If \(2\) marbles were randomly taken out of this sack at the same time, what is the probability that the \(2\) integers marked on the marbles taken out are coprime? [3 points]
  1. \(\dfrac{8}{21}\)
  2. \(\dfrac{10}{21}\)
  3. \(\dfrac{4}{7}\)
  4. \(\dfrac{2}{3}\)
  5. \(\dfrac{16}{21}\)

Mathematics (Type Ga)

Suppose \(0 \leq \theta < 2\pi\). The quadratic equation of \(x\),
\(6x^2 + (4\cos\theta)x + \sin\theta = 0\),
has no real solutions if and only if \(\alpha < \theta < \beta\). What is the value of \(3\alpha + \beta\,\)? [3 points]
  1. \(\dfrac{5}{6}\pi\)
  2. \(\pi\)
  3. \(\dfrac{7}{6}\pi\)
  4. \(\dfrac{4}{3}\pi\)
  5. \(\dfrac{3}{2}\pi\)
What is the number of ways to give \(8\) identical chocolates to \(4\) students \(\mathrm{A,B,C}\) and \(\mathrm{D}\) according to the following rules? [3 points]
  1. Each student receives at least \(1\) chocolate.
  2. Student \(\mathrm{A}\) receives more chocolates than student \(\mathrm{B}\).
  1. \(11\)
  2. \(13\)
  3. \(15\)
  4. \(17\)
  5. \(19\)

Mathematics (Type Ga)

In \(3\)-dimensional space, what is the \(x\)-coordinate of the point where a plane, containing the point \((2, 0, 5)\) and the line \(x-1 = 2-y = \dfrac{z+1}{2}\), meets the \(x\)-axis? [3 points]
  1. \(\dfrac{9}{2}\)
  2. \(4\)
  3. \(\dfrac{7}{2}\)
  4. \(3\)
  5. \(\dfrac{5}{2}\)
The graph of a quadratic function \(f(x)\) and a linear function \(g(x)\) is as the figure shows. What is the sum of all positive integers \(x\) for which
\(\left(\!\dfrac{1}{2}\!\right)^{\!f(x)g(x)} \geq \left(\!\dfrac{1}{8}\!\right)^{\!g(x)}\)
is true? [4 points]
  1. \(7\)
  2. \(9\)
  3. \(11\)
  4. \(13\)
  5. \(15\)

Mathematics (Type Ga)

On some day, the commuting time of employees of some company followed a normal distribution with a mean of \(66.4\) minutes and a standard deviation of
\(15\) minutes. On this day, \(40\%\) of employees whose commuting time was not less than \(73\) minutes, and \(20\%\) of employees whose commuting time was less than \(73\) minutes, commuted by the subway. All other employees commuted by other means. Among the employees of this company who commuted this day, what is the probability that a randomly selected employee has commuted by the subway?
(※ For a random variable \(Z\) that follows the standard normal distribution, suppose \(\mathrm{P}(0 \leq Z \leq 0.44) = 0.17\).) [4 points]
  1. \(0.306\)
  2. \(0.296\)
  3. \(0.286\)
  4. \(0.276\)
  5. \(0.266\)
A continuous function \(f(x)\) defined on \(x > 0\) satisfies
\(2f(x) + \dfrac{1}{x^2} f\left(\dfrac{1}{x}\right) = \dfrac{1}{x} + \dfrac{1}{x^2}\)
for all positive numbers \(x\).
What is the value of \(\displaystyle\int_\frac{1}{2}^2 \! f(x)dx\)? [4 points]
  1. \(\dfrac{\ln 2}{3} + \dfrac{1}{2}\)
  2. \(\dfrac{2\ln 2}{3} + \dfrac{1}{2}\)
  3. \(\dfrac{\ln 2}{3} + 1\)
  4. \(\dfrac{2\ln 2}{3} + 1\)
  5. \(\dfrac{2\ln 2}{3} + \dfrac{3}{2}\)

Mathematics (Type Ga)

Consider the set \(X = \{1,2,3,4,5,6\}\) and a function \(f: X \to X\). The following passage computes the number of functions \(f\) for which the image of the composite function \(f \circ f\) has \(5\) elements.
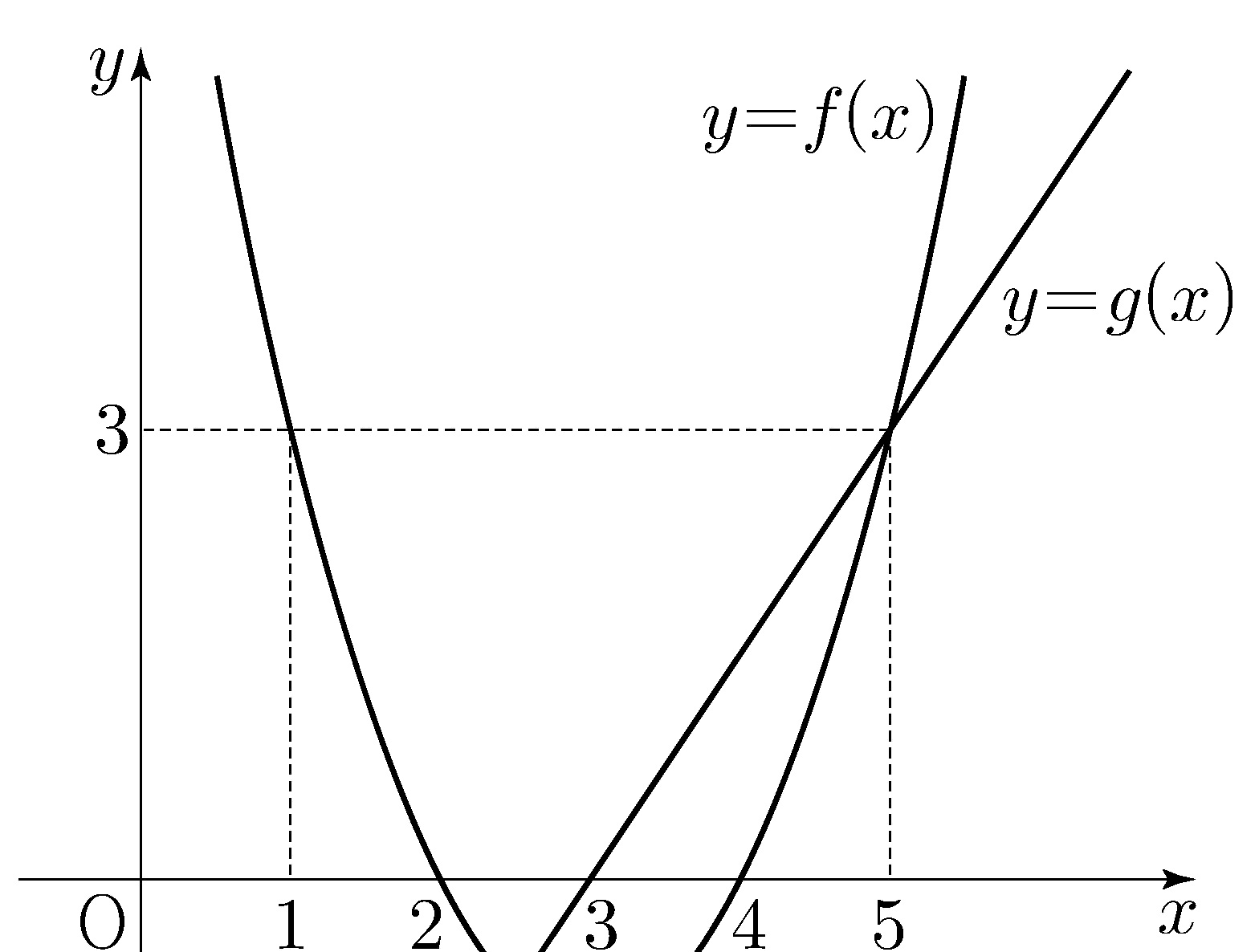
Let \(A\) and \(B\) be the image of \(f\) and \(f \circ f\) respectively.
If \(n(A) = 6\) then \(f\) is bijective, and \(f \circ f\) is also bijective, so \(n(B) = 6\).
If \(n(A) \leq 4\) then \(n(B) \leq 4\) since \(B \subseteq A\).
Therefore it should be that \(n(A) = 5\) and \(B = A\).
  1. There are \(\fbox{\(\;(\alpha)\;\)}\) ways to select a subset \(A\) of \(X\) where \(n(A) = 5\).
  2. For \(A\) selected in (i), let \(k\) be the element of \(X\) that is not an element of \(A\). Since \(n(A) = 5\), there are \(\fbox{\(\;(\beta)\;\)}\) ways to select \(f(k)\) from \(A\).
  3. For \(A=\{a_1,a_2,a_3,a_4,a_5\}\) selected in (i) and \(f(k)\) selected in (ii),
    since \(f(k) \in A\) and \(A = B\), it should be that \(A=\{f(a_1),f(a_2),f(a_3),f(a_4),f(a_5)\}\).
    The number of cases where this holds is equal to the number of bijections from \(A\) to \(A\), which is \(\fbox{\(\;(\gamma)\;\)}\).
By (i), (ii) and (iii), the number of functions \(f\) to compute is \(\fbox{\(\;(\alpha)\;\)}\times\fbox{\(\;(\beta)\;\)}\times\fbox{\(\;(\gamma)\;\)}\).
Let \(p\), \(q\) and \(r\) be the correct number for \((\alpha)\), \((\beta)\) and \((\gamma)\) respectively. What is the value of \(p + q + r\)? [4 points]
  1. \(131\)
  2. \(136\)
  3. \(141\)
  4. \(146\)
  5. \(151\)
As the figure shows, a right triangle \(\mathrm{ABC}\) satisfies \(\overline{\mathrm{AB}} = 1\) and \(\angle \mathrm{B} = \dfrac{\pi}{2}\). Let \(\mathrm{D}\) be the point where a line that bisects \(\angle \mathrm{C}\) meets the line segment \(\mathrm{AB}\). Let \(\mathrm{E}\) be the point where a circle with center \(\mathrm{A}\) and radius \(\overline{\mathrm{AD}}\) meets the line segment \(\mathrm{AC}\).
For \(\angle \mathrm{A} = \theta\), let \(S(\theta)\) be the area of the sector \(\mathrm{ADE}\), and let \(T(\theta)\) be the area of the triangle \(\mathrm{BCE}\).
What is the value of \(\displaystyle\lim_{\theta\to 0+} \!\! \dfrac{\{S(\theta)\}^2}{T(\theta)}\)? [4 points]
  1. \(\dfrac{1}{4}\)
  2. \(\dfrac{1}{2}\)
  3. \(\dfrac{3}{4}\)
  4. \(1\)
  5. \(\dfrac{5}{4}\)

Mathematics (Type Ga)

There is a tetrahedron \(\mathrm{ABCD}\) whose face \(\mathrm{BCD}\) is an equilateral triangle with side lengths \(12\). Let \(\mathrm{H}\) be the perpendicular foot from point \(\mathrm{A}\) to plane \(\mathrm{BCD}\).
It is given that \(\mathrm{H}\) lies inside the triangle \(\mathrm{BCD}\),
the area of triangle \(\mathrm{CDH}\) is \(3\) times the triangle \(\mathrm{BCH}\),
the area of triangle \(\mathrm{DBH}\) is \(2\) times the triangle \(\mathrm{BCH}\),
and \(\overline{\mathrm{AH}} = 3\). Let \(\mathrm{M}\) be the midpoint of the line segment \(\mathrm{BD}\), and let \(\mathrm{Q}\) be the perpendicular foot from point \(\mathrm{A}\) to line \(\mathrm{CM}\). What is the length of the line segment \(\mathrm{AQ}\)? [4 points]
  1. \(\sqrt{11}\)
  2. \(2\sqrt{3}\)
  3. \(\sqrt{13}\)
  4. \(\sqrt{14}\)
  5. \(\sqrt{15}\)
Let us draw all tangent lines to the curve \(y=\sin x \, (x > 0)\) which passes through the point \(\left(\!-\dfrac{\pi}{2}, 0\!\right)\), and list all of the \(x\)-coordinates of the points of tangency in ascending order.
Let \(a_n\) be the \(n\)th number in this list. Which option only contains every statement in the <List> that is true for all positive integers \(n\)? [4 points]
  1. \(\tan a_n = a_n + \dfrac{\pi}{2}\)
  2. \(\tan a_{n+2} - \tan a_n > 2\pi\)
  3. \(a_{n+1} + a_{n+2} > a_n + a_{n+3}\)
  1. a
  2. a, b
  3. a, c
  4. b, c
  5. a, b, c

Mathematics (Type Ga)

A function \(f(x)\) differentiable on the set of all real numbers satisfies the following. What is the value of \(f(-1)\)? [4 points]
  1. \(2\left\{f(x)\right\}^2f'(x) = \left\{f(2x+1)\right\}^2f'(2x+1)\)
    for all real numbers \(x\).
  2. \(f\!\left(\!-\dfrac{1}{8}\!\right) = 1\) and \(f(6)=2\).
  1. \(\dfrac{\sqrt[3]{3}}{6}\)
  2. \(\dfrac{\sqrt[3]{3}}{3}\)
  3. \(\dfrac{\sqrt[3]{3}}{2}\)
  4. \(\dfrac{2\sqrt[3]{3}}{3}\)
  5. \(\dfrac{5\sqrt[3]{3}}{6}\)
Short Answer Questions
Compute \(_6\mathrm{P}_2 - \,_6\mathrm{C}_2\). [3 points]
Given that \(\tan \theta = 5\), compute \(\sec^2 \theta\). [3 points]

Mathematics (Type Ga)

Suppose a point \(\mathrm{P}\) is moving on the \(xy\)-plane, and its position \((x, y)\) at time \(t \, (t \geq 0)\) is equal to
\(x = 1 - \cos 4t \:\) and \(\: y = \dfrac{1}{4} \sin 4t\).
Compute the magnitude of acceleration of point \(\mathrm{P}\) when its speed is at its greatest. [3 points]
Compute \(\displaystyle\int_0^\pi \! x \cos(\pi - x)dx\). [3 points]
In some region, the daily leisure time of residents follows a normal distribution with a mean of \(m\) minutes and a standard deviation of \(\sigma\) minutes.
Suppose \(16\) residents were randomly sampled from this region, and their daily leisure time had a mean of \(75\) minutes. The \(95\%\) confidence interval for \(m\) computed with this sample is \(a \leq m \leq b\).
Suppose \(16\) residents were randomly sampled from this region again, and their daily leisure time had a mean of \(77\) minutes. The \(99\%\) confidence interval for \(m\) computed with this sample is \(c \leq m \leq d\).
Given that \(d - b = 3.86\), Compute the value of \(\sigma\).
(※ For a random variable \(Z\) that follows the standard normal distribution, suppose \(\mathrm{P}(|Z| \leq 2.58) = 0.99\).) [4 points]

Mathematics (Type Ga)

Let us throw a die once. Let \(A\) be the event where it lands on an odd number, and let \(B\) be the event where it lands on a factor of \(m\) for a positive integer \(m \leq 6\). Compute the sum of all \(m\) for which the two events \(A\) and \(B\) are independent. [4 points]
Consider the ellipse \(\dfrac{x^2}{49} + \dfrac{y^2}{33} = 1\) with two foci \(\mathrm{F}\) and \(\mathrm{F'}\). For a point \(\mathrm{P}\) on the circle \(x^2 + (y-3)^2 = 4\), let \(\mathrm{Q}\) be the intersection of line \(\mathrm{F'P}\) and the ellipse that has a positive \(y\)-coordinate. Compute the maximum value of \(\overline{\mathrm{PQ}} + \overline{\mathrm{FQ}}\). [4 points]

Mathematics (Type Ga)

Consider a triangle \(\mathrm{ABC}\) on the \(xy\)-plane with an area of \(9\). Let \(\mathrm{P}\), \(\mathrm{Q}\) and \(\mathrm{R}\) be points that move freely on line segments \(\mathrm{AB}\), \(\mathrm{BC}\) and \(\mathrm{CA}\) respectively.
The set of all points \(\mathrm{X}\) that satisfy
\(\overrightarrow{\mathrm{AX}} = \dfrac{1}{4} \Big( \overrightarrow{\mathrm{AP}} + \overrightarrow{\mathrm{AR}} \Big) + \dfrac{1}{2} \overrightarrow{\mathrm{AQ}}\)
is a region with an area of \(\dfrac{q}{p}\). Compute \(p+q\).
(※ \(p\) and \(q\) are positive integers that are coprime.) [4 points]
Consider the function \(g(x)=\dfrac{1}{2+\sin \! \big(f(x)\big)}\) where \(f(x)\) is a cubic function with a leading coefficient of \(6\pi\).
Let us list all \(\alpha \geq 0\) for which \(g(x)\) has a local maximum or a local minimum at \(x=\alpha\).
Let \(\alpha_1, \alpha_2, \alpha_3, \alpha_4, \alpha_5, \cdots\) be this list in ascending order. \(g(x)\) satisfies the following.
  1. \(\alpha_1 = 0 \:\) and \(\: g(\alpha_1) = \dfrac{2}{5}\).
  2. \(\dfrac{1}{g(\alpha_5)} = \dfrac{1}{g(\alpha_2)} + \dfrac{1}{2}\).
Given that \(g'\!\left(\! -\dfrac{1}{2} \!\right) = a\pi\), compute \(a^2\).
(※ \(0 < f(0) < \dfrac{\pi}{2}\)) [4 points]