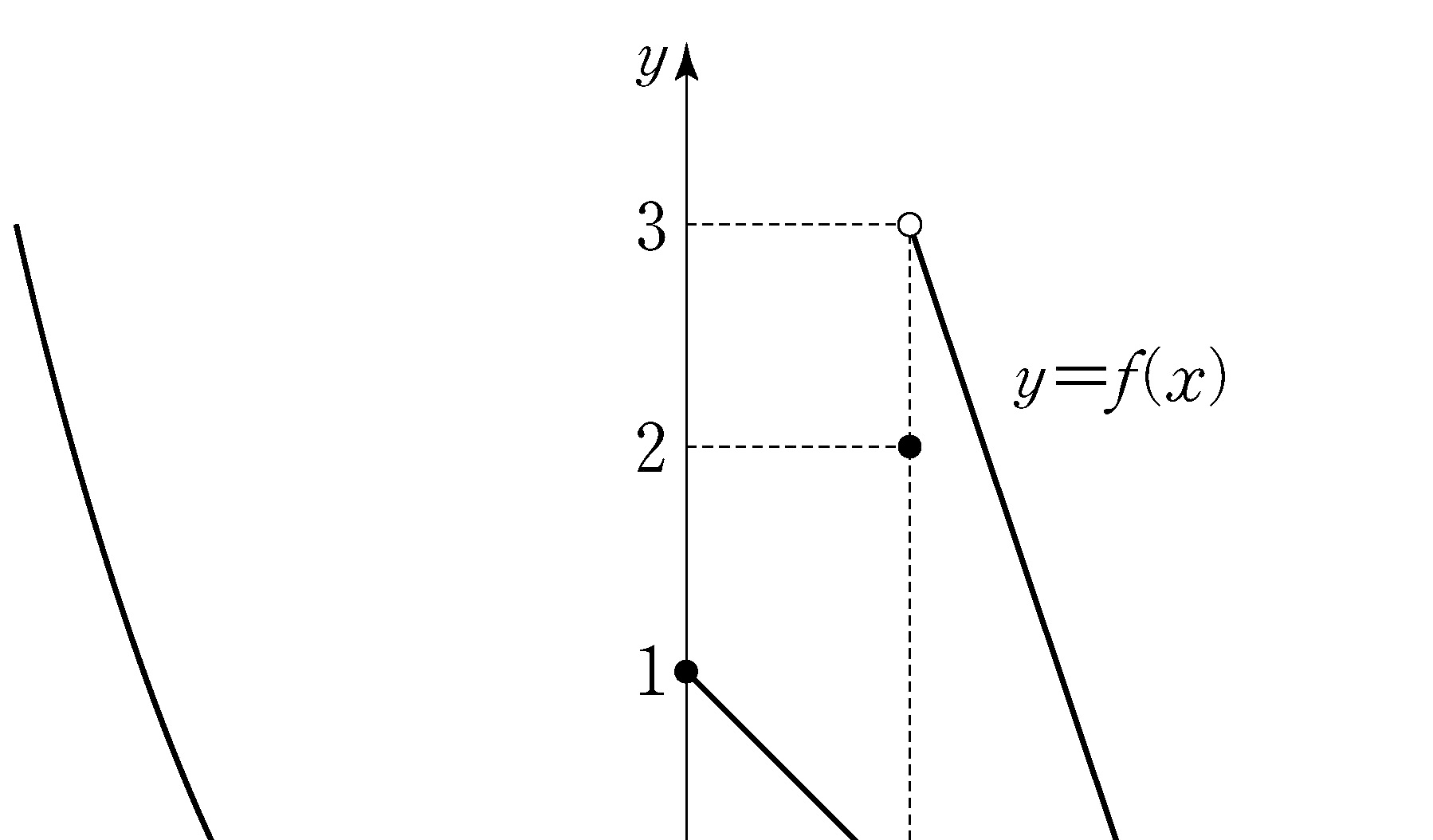
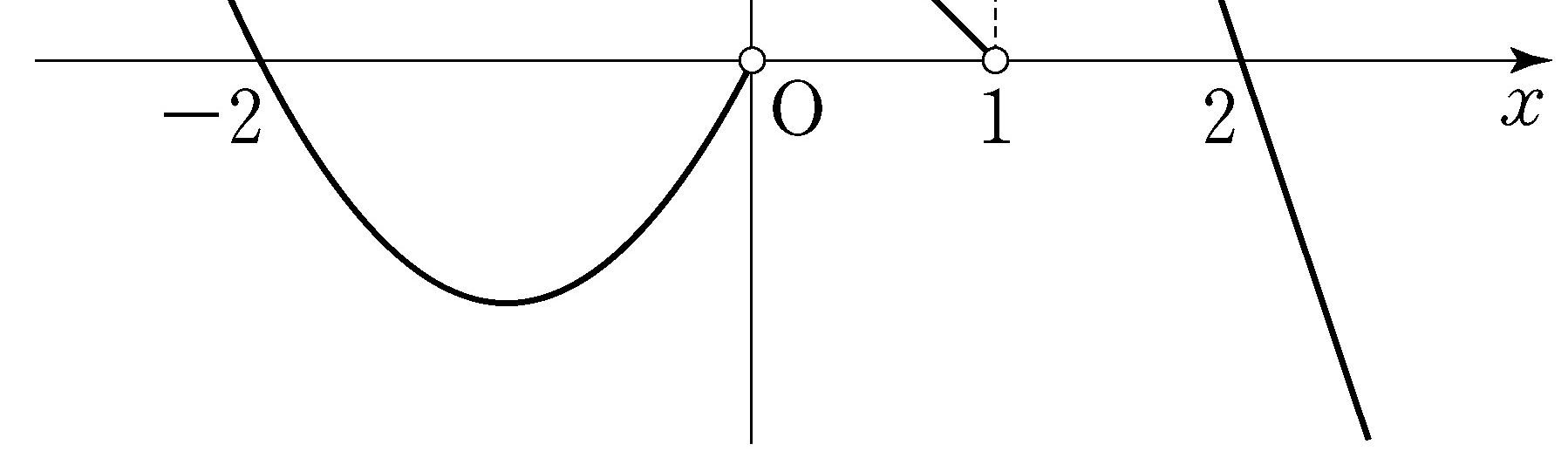
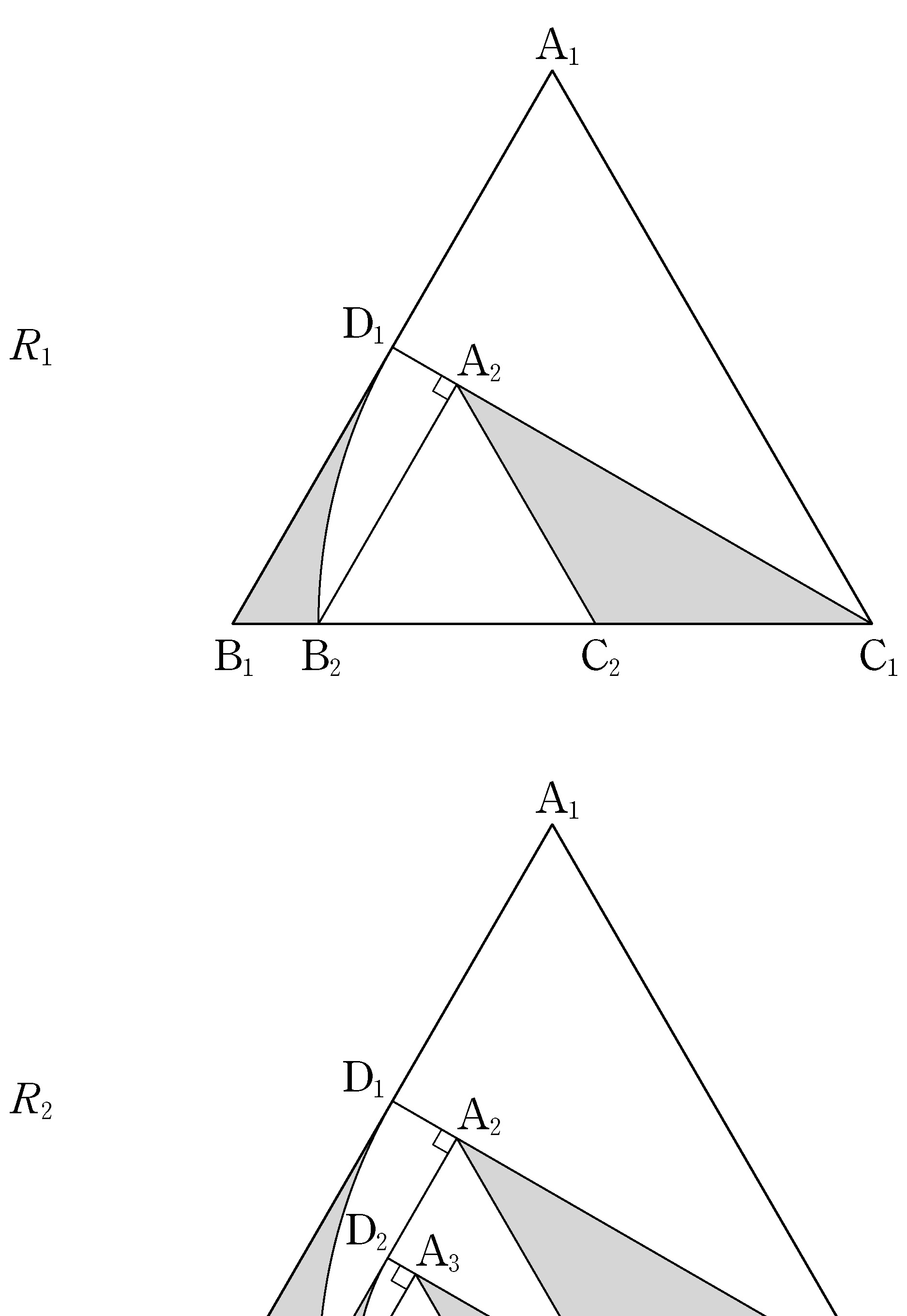
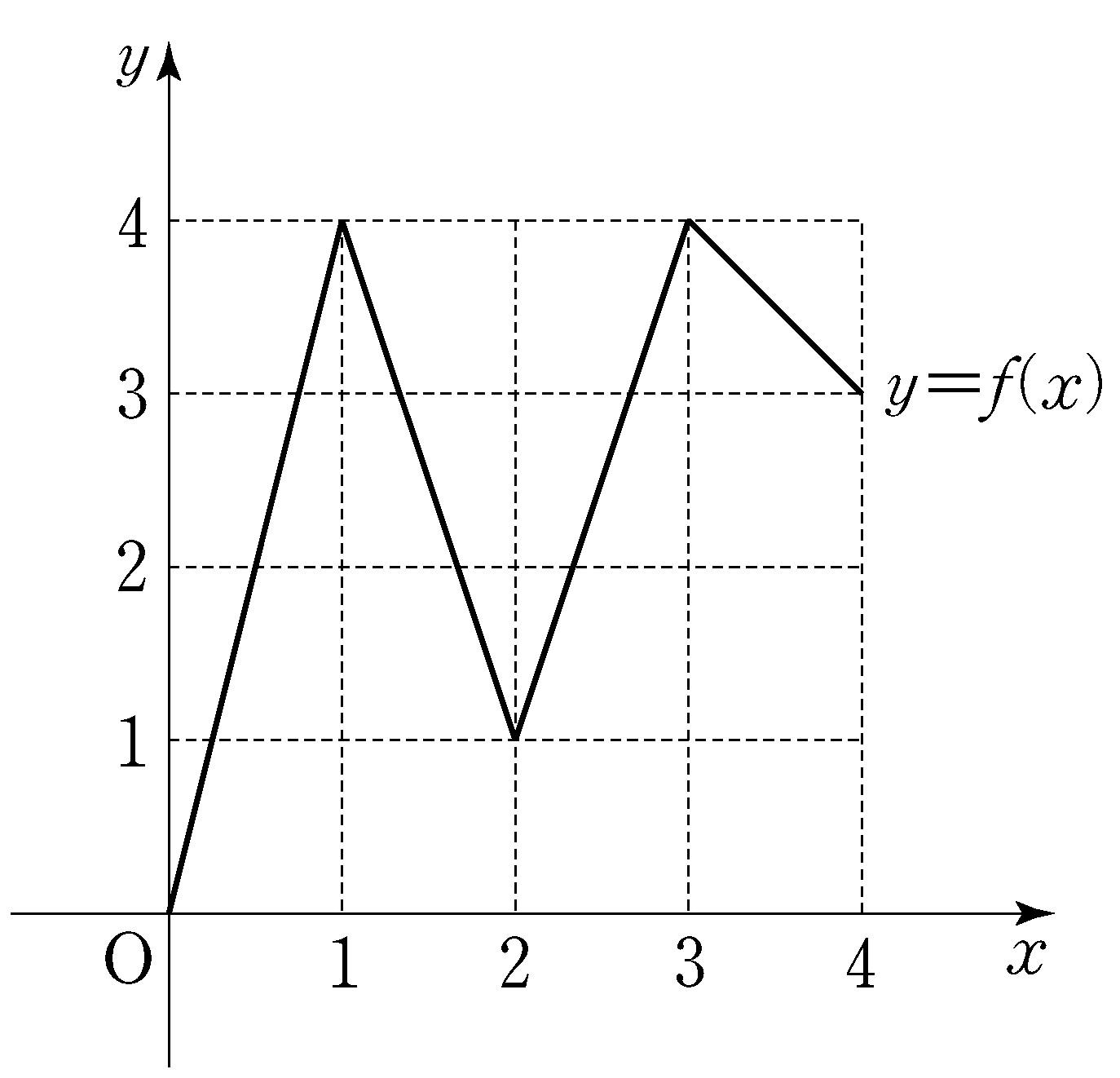
2018 College Scholastic Ability Test

Mathematics (Type Na)

Multiple Choice Questions
What is the value of \(2 \times 16^{^1/_4}\)? [2 points]
  1. \(2\)
  2. \(4\)
  3. \(6\)
  4. \(8\)
  5. \(10\)
Two sets
\(A=\{2,a+1,5\}\:\) and \(\:B=\{2,3,b\}\)
satisfy \(A=B\). What is the value of \(a+b\)? (※ \(a\) and \(b\) are real numbers.) [2 points]
  1. \(4\)
  2. \(5\)
  3. \(6\)
  4. \(7\)
  5. \(8\)
What is the value of \(\displaystyle\lim_{n\to\infty}\! \dfrac{5^n-3}{5^{n+1}}\)? [2 points]
  1. \(\dfrac{1}{5}\)
  2. \(\dfrac{1}{4}\)
  3. \(\dfrac{1}{3}\)
  4. \(\dfrac{1}{2}\)
  5. \(1\)
Figure below depicts two functions \(f: X \to Y\) and \(g: Y\to Z\).
What is the value of \((g \circ f)(2)\)? [3 points]
  1. \(1\)
  2. \(2\)
  3. \(3\)
  4. \(4\)
  5. \(5\)

Mathematics (Type Na)

Figure below is the graph of the function \(y=f(x)\).
What is the value of \(\displaystyle\lim_{x\to0-}\!f(x)+\lim_{x\to1+}\!f(x)\)? [3 points]
  1. \(1\)
  2. \(2\)
  3. \(3\)
  4. \(4\)
  5. \(5\)
Consider the following two conditions on a real number \(x\).
\(p : (x-1)(x-4)=0\),
\(q : 1<2x\leq a\)
What is the minimum value of the real number \(a\) for which \(p\) is sufficient for \(q\)? [3 points]
  1. \(4\)
  2. \(5\)
  3. \(6\)
  4. \(7\)
  5. \(8\)
A survey was conducted on all of the \(500\) students in some high school, about whether they want a regional culture tour on regions \(\mathrm{A}\) and \(\mathrm{B}\).
(Unit: people)
Region \(\mathrm{A}\)
Region \(\mathrm{B}\)
 Want Not want Total
Want \(140\) \(310\) \(450\)
Not want \(40\) \(10\) \(50\)
Total \(180\) \(320\) \(500\)
Given that a randomly selected student among the students of this high school wants region \(\mathrm{A}\), what is the probability that this student also wants region \(\mathrm{B}\)? [3 points]
  1. \(\dfrac{19}{45}\)
  2. \(\dfrac{23}{45}\)
  3. \(\dfrac{3}{5}\)
  4. \(\dfrac{31}{45}\)
  5. \(\dfrac{7}{9}\)

Mathematics (Type Na)

What is the number of integer partitions of \(11\) where each part is between \(3\) and \(7\), inclusive? [3 points]
  1. \(2\)
  2. \(4\)
  3. \(6\)
  4. \(8\)
  5. \(10\)
What is the value of the positive number \(a\) that satisfies \(\displaystyle\int_0^a\!(3x^2-4)dx=0\)? [3 points]
  1. \(2\)
  2. \(\dfrac{9}{4}\)
  3. \(\dfrac{5}{2}\)
  4. \(\dfrac{11}{4}\)
  5. \(3\)
Consider two events \(A\) and \(B\) that are independent. Given that
\(\mathrm{P}(A) = \dfrac{2}{3}\, \) and \(\, \mathrm{P}(A \cup B) = \dfrac{5}{6}\),
what is the value of \(\mathrm{P}(B)\)? [3 points]
  1. \(\dfrac{1}{3}\)
  2. \(\dfrac{5}{12}\)
  3. \(\dfrac{1}{2}\)
  4. \(\dfrac{7}{12}\)
  5. \(\dfrac{2}{3}\)

Mathematics (Type Na)

On the \(xy\)-plane, consider the region enclosed by the curve \(y=\dfrac{1}{2x-8}+3\), the \(x\)-axis, and the \(y\)-axis. What is the number of points inside this region with the \(x\)-coordinate and \(y\)-coordinate both being positive integers? [3 points]
  1. \(3\)
  2. \(4\)
  3. \(5\)
  4. \(6\)
  5. \(7\)
In the expansion of \(\left(\!x+\dfrac{2}{x}\!\right)^{\!8}\), what is the coefficient of \(x^4\)? [3 points]
  1. \(128\)
  2. \(124\)
  3. \(120\)
  4. \(116\)
  5. \(112\)

Mathematics (Type Na)

Consider a sequence \(\{a_n\}\) where \(a_1 = 2\), and the following is true for all positive integers \(n\).
\( a_{n+1} = \begin{cases} a_n-1 &\; (\text{if } a_n \text{ is even})\\ \\ a_n+n &\; (\text{if } a_n \text{ is odd}) \end{cases} \)
What is the value of \(a_7\)? [3 points]
  1. \(7\)
  2. \(9\)
  3. \(11\)
  4. \(13\)
  5. \(15\)
An arithmetic progression \(\{a_n\}\) satisfies
\(a_5+a_{13}=3a_9\:\) and \(\:\displaystyle\sum_{k=1}^{18}a_k=\frac{9}{2}\).
What is the value of \(a_{13}\)? [4 points]
  1. \(2\)
  2. \(1\)
  3. \(0\)
  4. \(-1\)
  5. \(-2\)

Mathematics (Type Na)

The weight of a cosmetic product manufactured in some company follows a normal distribution with a mean of \(201.5\text{g}\) and a standard deviation of \(1.8\text{g}\). What is the probability that \(9\) products randomly
sampled from the cosmetic products manufactured in this company, have a sample mean of \(200\text{g}\) or more, computed using the standard normal table to the right? [4 points]
\(z\)\(\mathrm{P}(0\!\leq\! Z \!\leq\!z)\)
\(1.0\)\(0.3413\)
\(1.5\)\(0.4332\)
\(2.0\)\(0.4772\)
\(2.5\)\(0.4938\)
  1. \(0.7745\)
  2. \(0.8413\)
  3. \(0.9332\)
  4. \(0.9772\)
  5. \(0.9938\)
Two real numbers \(a\) and \(b\) both greater than \(1\), satisfy
\(\log_{\sqrt{3}}a=\log_9 ab\).
What is the value of \(\log_a b\)? [4 points]
  1. \(1\)
  2. \(2\)
  3. \(3\)
  4. \(4\)
  5. \(5\)

Mathematics (Type Na)

The probability distribution of a random variable \(X\) is as follows.
\(X\) \(0.121\) \(0.221\) \(0.321\) Total
\(\mathrm{P}(X=x)\) \(a\) \(b\) \(\dfrac{2}{3}\) \(1\)
The following is a process computing \(\mathrm{V}(X)\), given that \(\mathrm{E}(X)=0.271\).
Let \(Y=10X-2.21\). The probability distribution of the random variable \(Y\) is as follows.
\(Y\) \(-1\) \(0\) \(1\) Total
\(\mathrm{P}(Y=y)\) \(a\) \(b\) \(\dfrac{2}{3}\) \(1\)
Since \(\:\mathrm{E}(Y)=10\,\mathrm{E}(X)-2.21=0.5\),
\(a=\fbox{\(\;(\alpha)\;\)}\,,\;b=\fbox{\(\;(\beta)\;\)}\,,\)
and \(\mathrm{V}(Y)=\dfrac{7}{12}\).
Since \(Y=10X-2.21,\;\mathrm{V}(Y)=\fbox{\(\;(\gamma)\;\)}\times\mathrm{V}(X)\).
Therefore \(\mathrm{V}(X)=\dfrac{1}{\fbox{\(\;(\gamma)\;\)}}\times\dfrac{7}{12}\).
Let \(p, q\) and \(r\) be the correct number for \((\alpha), (\beta)\) and \((\gamma)\) respectively. What is the value of \(pqr\)? [4 points]
  1. \(\dfrac{13}{9}\)
  2. \(\dfrac{16}{9}\)
  3. \(\dfrac{19}{9}\)
  4. \(\dfrac{22}{9}\)
  5. \(\dfrac{25}{9}\)
A cubic function \(f(x)\) with a leading coefficient of \(1\) satisfies \(f(1)=0\) and
\(\displaystyle\lim_{x\to 2}\frac{f(x)}{(x-2)\{f'(x)\}^2}=\frac{1}{4}\).
What is the value of \(f(3)\)? [4 points]
  1. \(4\)
  2. \(6\)
  3. \(8\)
  4. \(10\)
  5. \(12\)

Mathematics (Type Na)

As the figure shows, there is an equilateral triangle \(\mathrm{A_1B_1C_1}\) with side lengths of \(1\). Let \(\mathrm{D_1}\) be the midpoint of the line segment \(\mathrm{A_1B_1}\). For a point \(\mathrm{B_2}\) on the line segment \(\mathrm{B_1C_1}\) satisfying \(\overline{\mathrm{C_1D_1}}=\overline{\mathrm{C_1B_2}}\), let us draw the sector \(\mathrm{C_1D_1B_2}\) with center \(\mathrm{C_1}\). Let \(\mathrm{A_2}\) be the perpendicular foot from point \(\mathrm{B_2}\) to line \(\mathrm{C_1D_1}\), and let \(\mathrm{C_2}\) be the midpoint of the line segment \(\mathrm{C_1B_2}\). Figure \(R_1\) is obtained by coloring inside the triangle \(\mathrm{C_1A_2C_2}\), and the region enclosed by arc \(\mathrm{D_1B_2}\) and lines \(\mathrm{B_1B_2}\) and \(\mathrm{B_1D_1}\).
Starting from figure \(R_1\), let \(\mathrm{D_2}\) be the midpoint of the line segment \(\mathrm{A_2B_2}\). For a point \(\mathrm{B_3}\) on the line segment \(\mathrm{B_2C_2}\) satisfying \(\overline{\mathrm{C_2D_2}}=\overline{\mathrm{C_2B_3}}\), let us draw the sector \(\mathrm{C_2D_2B_3}\) with center \(\mathrm{C_2}\). Let \(\mathrm{A_3}\) be the perpendicular foot from point \(\mathrm{B_3}\) to line \(\mathrm{C_2D_2}\), and let \(\mathrm{C_3}\) be the midpoint of the line segment \(\mathrm{C_2B_3}\). Figure \(R_2\) is obtained by coloring inside the triangle \(\mathrm{C_2A_3C_3}\), and the region enclosed by arc \(\mathrm{D_2B_3}\) and lines \(\mathrm{B_2B_3}\) and \(\mathrm{B_2D_2}\).
Continue this process, and let \(S_n\) be the area of the colored region in \(R_n\), the \(n\)th obtained figure.
What is the value of \(\displaystyle\lim_{n\to\infty} S_n\)? [4 points]
  1. \(\dfrac{11\sqrt{3}-4\pi}{56}\)
  2. \(\dfrac{11\sqrt{3}-4\pi}{52}\)
  3. \(\dfrac{15\sqrt{3}-6\pi}{56}\)
  4. \(\dfrac{15\sqrt{3}-6\pi}{52}\)
  5. \(\dfrac{15\sqrt{3}-4\pi}{52}\)
A quartic function \(f(x)\) with a leading coefficient of \(1\) satisfies the following.
  1. \(f'(0)=0\:\) and \(\:f'(2)=16\).
  2. \(f'(x)<0\) on intervals \((-\infty,0)\) and \((0,k)\), where \(k\) is some positive number.
Which option only contains every correct statement in the <List>? [4 points]
  1. The equation \(f'(x)=0\) has one solution on the open interval \((0,2)\).
  2. The function \(f(x)\) has a local maximum.
  3. If \(f(0)=0\), then \(f(x)\geq -\dfrac{1}{3}\) for all real numbers \(x\).
  1. a
  2. b
  3. a, c
  4. b, c
  5. a, b, c

Mathematics (Type Na)

As the figure shows, a function \(f(x)\) is defined on the closed interval \([0,4]\), and its graph is the same as the points \((0,0),\:(1,4),\:(2,1),\:(3,4)\) and \((4,3)\) connected with line segments in this order.
What is the number of sets \(X=\{a,b\}\) that satisfy the following? (※ \(0\leq a<b\leq 4\)) [4 points]
There exists a function \(g(x)=f(f(x))\) from \(X\) to \(X\), where \(g(a)=f(a)\) and \(g(b)=f(b)\).
  1. \(11\)
  2. \(13\)
  3. \(15\)
  4. \(17\)
  5. \(19\)
Short Answer Questions
Compute \(_5\mathrm{C}_3\). [3 points]
For the function \(f(x)=2x^3+x+1\), compute \(f'(1)\). [3 points]

Mathematics (Type Na)

For the universe \(\,U=\{1,2,3,4,5,6,7,8\}\) and its two subsets
\(A=\{1,2,3\}\:\) and \(\:B=\{2,4,6,8\}\),
compute \(n(A\cup B^{\,C}).\) [3 points]
A function \(f(x)\) satisfies \(\displaystyle\lim_{x\to1}(x+1)f(x)=1\). Given that \(\displaystyle\lim_{x\to1}(2x^2+1)f(x)=a\), compute \(20a\). [3 points]
The region enclosed by the curve \(y=-2x^2+3x\) and the line \(y=x\) has an area of \(\dfrac{q}{p}\). Compute \(p+q\). (※ \(p\) and \(q\) are positive integers that are coprime.) [4 points]

Mathematics (Type Na)

A sequence \(\{a_n\}\) satisfies
\(\displaystyle\sum_{k=1}^{10}(a_k+1)^2=28\:\) and \(\:\displaystyle\sum_{k=1}^{10}a_k(a_k+1)=16\).
Compute \(\displaystyle\sum_{k=1}^{10}(a_k)^2\). [4 points]
Suppose we throw a coin \(6\) times. The probability that the number of times it lands on heads is greater than the number of times it lands on tails, is \(\dfrac{q}{p}\). Compute \(p+q\).
(※ \(p\) and \(q\) are positive integers that are coprime.) [4 points]

Mathematics (Type Na)

For two real numbers \(a\) and \(k\), functions \(f(x)\) and \(g(x)\) are defined as
\(f(x)=\begin{cases} 0 & (x\leq a)\\\\ (x-1)^2(2x+1) & (x>a) \end{cases}\)
and
\(g(x)=\begin{cases} 0 & (x\leq k)\\\\ 12(x-k) & (x>k), \end{cases}\)
and satisfy the following.
  1. The function \(f(x)\) is differentiable on the set of all real numbers.
  2. \(f(x)\geq g(x)\) for all real numbers \(x\).
Given that the smallest possible value for \(k\) is \(\dfrac{q}{p}\), compute \(a+p+q\).
(※ \(p\) and \(q\) are positive integers that are coprime.) [4 points]
For the quadratic function \(f(x)=\dfrac{3x-x^2}{2}\), a function \(g(x)\) defined on the interval \([0,\infty)\) satisfies the following.
  1. For \(0\leq x<1\), \(g(x)=f(x)\).
  2. For \(n\leq x<n+1\),
    \(g(x)=\dfrac{1}{2^n}\{f(x-n)-(x-n)\}+x\).
    (※ This holds for all positive integers \(n\).)
For some positive integer \(k\,(k\geq 6)\), a function \(h(x)\) is defined as
\(h(x)=\begin{cases} g(x) & (0\leq x<5\: \text{ or } \:x\geq k)\\\\ 2x-g(x) & (5\leq x< k). \end{cases}\)
For a sequence \(\{a_n\}\) defined as \(a_n=\displaystyle\int_0^n h(x)dx\),
it is given that \(\displaystyle\lim_{n\to\infty}\!\big(2a_n-n^2\big)=\frac{241}{768}.\) Compute \(k\). [4 points]