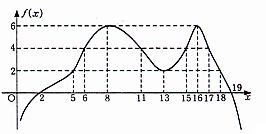
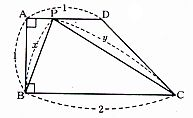
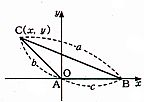
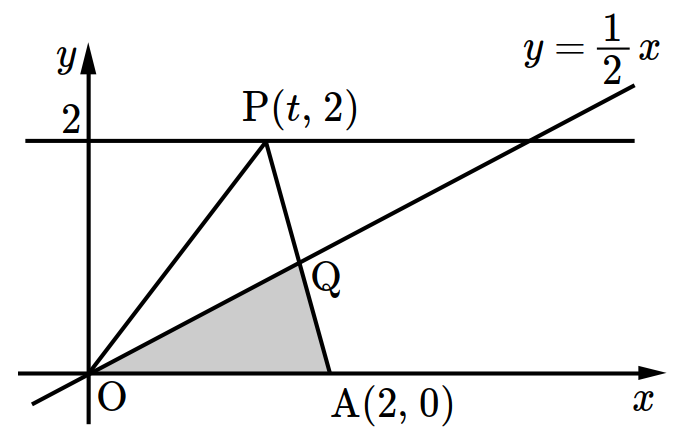
1995 College Scholastic Ability Test

Mathematics·Studies (I)

Nat. Sciences
Let \(\alpha\) and \(\beta\) be the two solutions to the quadratic equation \(2x^2-4x-1=0\). What is the value of \(\alpha^3 + \beta^3\)? [1 point]
  1. \(1\)
  2. \(3\)
  3. \(4\)
  4. \(8\)
  5. \(11\)
Let \(\alpha\) be the solution to the equation \(3^{x+2}=96\). Which option below is correct? [1 point]
  1. \(0 < \alpha < 1\)
  2. \(1 < \alpha < 2\)
  3. \(2 < \alpha < 3\)
  4. \(3 < \alpha < 4\)
  5. \(4 < \alpha < 5\)
\(2\times 2\) matrices \(A\) and \(B\) are as follows.
\(A=\begin{pmatrix*}[r] 2&-4 \\ -1&2 \end{pmatrix*},\; B=\begin{pmatrix} 1&2 \\ 2&4 \end{pmatrix}\)
Which option is equal to the matrix \(\dfrac{1}{3}AB-BA\)? [1 point]
  1. \(\begin{pmatrix*}[r] -2&-4 \\ 1&2 \end{pmatrix*}\)
  2. \(\begin{pmatrix*}[r] -2&8 \\ 2&-4 \end{pmatrix*}\)
  3. \(\begin{pmatrix*}[r] -4&-8 \\ 2&4 \end{pmatrix*}\)
  4. \(\begin{pmatrix*}[r] -6&-12 \\ 3&6 \end{pmatrix*}\)
  5. \(\begin{pmatrix*}[r] 0&0 \\ 0&0 \end{pmatrix*}\)
What is the value of the integral \(\displaystyle\int_{0}^{\pi}(1-\cos^3 x)\cos x\sin x\,dx\)? [1 point]
  1. \(0\)
  2. \(-\dfrac{1}{5}\)
  3. \(-\dfrac{2}{5}\)
  4. \(-\dfrac{3}{5}\)
  5. \(-\dfrac{4}{5}\)

Mathematics·Studies (I)

Nat. Sciences
For the universe \(U\) and its two subsets \(A\) and \(B\), given that \(A \subseteq B\), which option below is not always true? (※ \(U\ne\varnothing\)) [1 point]
  1. \(A\cup B=B\)
  2. \(A\cap B=A\)
  3. \((A\cap B)^c=B^c\)
  4. \(B^c \subseteq A^c\)
  5. \(A-B=\varnothing\)
Let \(f(x)=2x-1\). A function \(g(x)\) satisfies
\((h \circ g \circ f)(x)=h(x)\)
for all functions \(h(x)\). What is the value of \(g(3)\)?
(※ \(f(x), g(x)\) and \(h(x)\) are functions from \(R\) to \(R\), where \(R\) is the set of all real numbers.) [1 point]
  1. \(-2\)
  2. \(-1\)
  3. \(0\)
  4. \(1\)
  5. \(2\)
As the figure shows, there are \(7\) points on a semicircle. What is the number of triangles that have three of these points as its vertices? [1 point]
  1. \(34\)
  2. \(33\)
  3. \(32\)
  4. \(31\)
  5. \(30\)
Suppose
\(\dfrac{1}{(x-1)(x-2)\cdots(x-10)}\)
\(=\dfrac{a_1}{x-1} + \dfrac{a_2}{x-2} + \cdots + \dfrac{a_{10}}{x-10}\)
is satisfied for all real numbers \(x\) that does not make the denominator \(0\). What is the value of \(a_1+a_2+\cdots+a_{10}\)? [1.5 points]
  1. \(0\)
  2. \(-1\)
  3. \(1\)
  4. \(-10\)
  5. \(10\)

Mathematics·Studies (I)

Nat. Sciences
\(x\) and \(y\) are real numbers that satisfy \((x+y)(x-y)\ne 0\) and
\(\sqrt{\dfrac{x+y}{x-y}}=-\dfrac{\sqrt{x+y}}{\sqrt{x-y}}\).
Which option below depicts the region on the \(xy\)-plane where the point \((x, y)\) can exist? (※ The dotted lines are not included.) [1 point]
Consider a function \(f(x)\) that is continuous, but not differentiable, at \(x=0\). Which option contains every function in the <List> that is differentiable at \(x=0\)? [1.5 points]
  1. \(y=xf(x)\)
  2. \(y=x^2f(x)\)
  3. \(y=\dfrac{1}{1+xf(x)}\)
  1. a
  2. b
  3. c
  4. a, b
  5. a, b, c
A point \(\mathrm{P}\) starts from the origin and moves on the number line for \(7\) seconds, and its velocity \(v(t)\) at time \(t\) is as the figure shows. Which option contains every correct statement in the <List>? [1.5 points]
  1. After point \(\mathrm{P}\) started moving, there was a moment where it stopped for \(1\) second.
  2. While point \(\mathrm{P}\) was moving, it changed its direction \(4\) times.
  3. Point \(\mathrm{P}\) was on the starting point
    \(4\) seconds after it started moving.
  1. a
  2. c
  3. a, b
  4. a, c
  5. b, c
† Rephrasing statement a.: “Between \(\boldsymbol{0\leq t \leq 7}\), there was a moment where point \(\mathrm{P}\) stopped for \(1\) second.”
For all probability density functions \(f(x)\) and \(g(x)\) defined on the closed interval \([0,1]\), which option below can be a probability density function? [1 point]
  1. \(f(x)-g(x)\)
  2. \(f(x)+g(x)\)
  3. \(\dfrac{1}{2}\{f(x)-g(x)\}\)
  4. \(\dfrac{1}{3}\{2f(x)+g(x)\}\)
  5. \(2f(x)-g(x)\)

Mathematics·Studies (I)

Nat. Sciences
What is the number of complex numbers \(\alpha\) for which the value of \(|z-\alpha|\) is constant for all complex numbers \(z\) that satisfy \(|z|=1\)? [1.5 points]
  1. \(1\)
  2. \(2\)
  3. \(3\)
  4. \(4\)
  5. Infinitely many.
What is the value of \(m, n\) and \(k\) printed by the following flowchart, respectively? [1.5 points]
  1. \(0,2\) and \(5\)
  2. \(0,2\) and \(6\)
  3. \(0,5\) and \(3\)
  4. \(2,3\) and \(6\)
  5. \(2,3\) and \(5\)
Consider two points \(\mathrm{O}(0,0,0)\) and \(\mathrm{A}(1,0,0)\), and a point \(\mathrm{P}(x,y,z)\) that moves in \(3\)-dimensional space such that the area of \(\triangle \mathrm{OAP}\) is \(2\). Given that \(0\leq x\leq 1\), consider the shape created by the locus of point \(\mathrm{P}\). What is the area of this shape when spread on a plane? [1.5 points]
  1. \(16\pi\)
  2. \(8\pi\)
  3. \(5\pi\)
  4. \(2\pi\)
  5. \(\pi\)
Consider a right triangle \(\mathrm{ABC}\) where \(\angle \mathrm{C}\) is a right angle and the magnitude of \(\angle \mathrm{B}\) is \(\dfrac{\pi}{3}\). Let \(\mathrm{D}\) be a point on the edge \(\mathrm{BC}\), and let \(\theta\) be the magnitude of \(\angle \mathrm{BAD}\). What is \(\dfrac{\:\overline{\mathrm{BD}}\:}{\overline{\mathrm{AB}}}\) written as a function on \(\theta\)? [1.5 points]
  1. \(\sin\theta\)
  2. \(\dfrac{\sin\theta}{1+\cos\theta}\)
  3. \(\dfrac{2\sin\theta}{1+2\cos\theta}\)
  4. \(\dfrac{2\sin\theta}{\sin\theta+\sqrt{3}\cos\theta}\)
  5. \(\dfrac{1-\cos\theta}{2}\)

Mathematics·Studies (I)

Nat. Sciences
An object starts from the origin and moves along a spiral shown to the right, with a constant speed. Let \(x(t)\) be the \(x\)-coordinate of this object at time \(t\). Which option below is an appropriate graph of the relation between \(t\) and \(x(t)\)? [1 point]
Figure below shows the graph of the function \(y=f(x)\). Which option below lists the number of distinct real solutions, and the sum of distinct real solutions, of the equation \(f(f(x+2))=4\) solved for \(x\)? (※ \(f(x)<0\) when \(x<2\) or \(x>19\).) [1.5 points]
  1. \(2,20\)
  2. \(2,22\)
  3. \(3,30\)
  4. \(4,42\)
  5. \(4,50\)
Let us represent a positive integer \(n\) as \(n=2^p \cdot k\)
(\(p\) is a nonnegative integer, \(k\) is an odd number), and let \(f(n)=p\). For example, \(f(12)=2\). Which option contains every correct statement in the <List>? [1 point]
  1. If \(n\) is an odd number, then \(f(n)=0\).
  2. \(f(8)<f(24)\).
  3. There are infinitely many positive integers \(n\) that satisfy \(f(n)=3\).
  1. a
  2. b
  3. a, b
  4. a, c
  5. b, c
Let the set \(U=\{1,2,3,4,\cdots,100\}\). Among sets \(A\) that are a subset of \(U\) and satisfies the following conditions (A) and (B), what is the set with the smallest number of elements? [1 point]
  1. \(3\in A\)
  2. If \(m, n\in A\) and \(m+n \in U\), then \(m+n \in A\).
  1. \(A=\{3,9,15,21,\cdots,99\}\)
  2. \(A=\{3,6,9,12,\cdots,99\}\)
  3. \(A=\{3,4,5,6,\cdots,100\}\)
  4. \(A=\{1,3,5,7,\cdots,99\}\)
  5. \(A=\{1,2,3,4,\cdots,100\}\)

Mathematics·Studies (I)

Nat. Sciences
As the figure shows, there is a trapezoid \(\mathrm{ABCD}\).
\(\overline{\mathrm{AB}}=\overline{\mathrm{AD}}=1\), \(\:\overline{\mathrm{BC}}=2\),
and the magnitude of \(\angle \mathrm{A}\) and \(\angle \mathrm{B}\) are \(\dfrac{\pi}{2}\).
Let \(\mathrm{P}\) be a point on the top edge \(\mathrm{AD}\) and let \(\overline{\mathrm{PB}}=x\) and \(\overline{\mathrm{PC}}=y\). Which option contains every correct statement in the <List>? [1.5 points]
  1. \(xy\geq 2\).
  2. If \(xy=2\), then \(\triangle \mathrm{BCP}\) is a right triangle.
  3. \(xy\leq \sqrt{5}\).
  1. a
  2. c
  3. a, c
  4. b, c
  5. a, b, c
The following is a proof of the law of cosines relating the side lengths of a triangle to the cosine of angles, specifically if \(\angle \mathrm{A}\) is obtuse in \(\triangle \mathrm{ABC}\).
(Proof) As the figure shows, let \(\triangle \mathrm{ABC}\) with side lengths \(a, b\) and \(c\) be on the \(xy\)-plane so that point \(\mathrm{A}\) is on the origin. Let \((x,y)\) be the coordinates of point \(\mathrm{C}\). Then
\(x= \fbox{\(\;(\alpha)\;\)}\) and \(y= \fbox{\(\;(\beta)\;\)}\),
so the following holds by the Pythagorean theorem.
\(\begin{align}a^2 &= (\fbox{\(\;(\gamma)\;\)})^2+y^2\\ &= b^2+c^2-2bc \cos A \end{align}\)
In the proof above, what are appropriate for \((\alpha), (\beta)\) and \((\gamma)\) in this order? [1 point]
① \(b\cos A\), \(b\sin A\), \(c+x\)
② \(b\cos A\), \(b\sin A\), \(c-x\)
③ \(b\cos A\), \(-b\sin A\), \(c+x\)
④ \(-b\cos A\), \(-b\sin A\), \(c-x\)
⑤ \(-b\cos A\), \(-b\sin A\), \(c+x\)
For a cubic equation \(x^3+ax^2+bx+c=0\) with three real solutions, let \(\alpha, \beta\) and \(\gamma\) be the three solutions. The following is a proof that at least one of the three solutions has an absolute value greater than or equal to \(\dfrac{|a|}{3}\).
(Proof)
Let us assume the conclusion is false and \(\fbox{ (A) }\). Then
\(|\,\alpha\,|< \dfrac{|a|}{3},\; |\,\beta\,|< \dfrac{|a|}{3}\:\) and \(\:|\,\gamma\,|< \dfrac{|a|}{3}\).
Using the relation between solutions and coefficients,
\(a= \fbox{ (B) }\),
and
\(\begin{align} |a| &\leq |\alpha+\beta| + |\gamma| \\ &\leq \fbox{ (C) }\\ &< \dfrac{|a|}{3}+\dfrac{|a|}{3}+\dfrac{|a|}{3}=|a|. \end{align}\)
However, this contradicts the premise. Therefore at least one of the three solutions has an absolute value greater than or equal to \(\dfrac{|a|}{3}\).
In the proof above, what are appropriate for \(\text{(A)}, \text{(B)}\) and \(\text{(C)}\) in this order? [1 point]
  1. some solutions have an absolute value less than \(\dfrac{|a|}{3}\),
    \(-(\alpha+\beta+\gamma),\quad |\alpha|+|\beta|+|\gamma|\)
  2. some solutions have an absolute value less than or equal to \(\dfrac{|a|}{3}\),
    \(\alpha+\beta+\gamma,\quad |\alpha|+|\beta|+|\gamma|\)
  3. all solutions have an absolute value less than \(\dfrac{|a|}{3}\),
    \(\alpha+\beta+\gamma,\quad |\alpha+\beta+\gamma|\)
  4. some solutions have an absolute value less than \(\dfrac{|a|}{3}\),
    \(-(\alpha+\beta+\gamma),\quad |\alpha|+|\beta|+|\gamma|\)
  5. all solutions have an absolute value less than or equal to \(\dfrac{|a|}{3}\),
    \(\alpha+\beta+\gamma,\quad |\alpha+\beta+\gamma|\)

Mathematics·Studies (I)

Nat. Sciences
The following is a proof of a theorem about the harmonic mean.
(Proof) For positive numbers \(a,b\) and \(H\), suppose a real number \(r\) exists such that
\(a=H+\dfrac{a}{r}\) and \(H=b+\dfrac{b}{r}\)
\((A)\)
is satisfied. Then \(a\ne b\) and
\(\dfrac{a-H}{a}= \fbox{\(\;(\alpha)\;\)}\)
\((B)\)
therefore \(H=\fbox{\(\;(\beta)\;\)}\).
Conversely, for positive numbers \(a\) and \(b\) where \(a\ne b\), if \(H=\fbox{\(\;(\beta)\;\)}\), then the expression \((B)\) holds and \(\dfrac{a-H}{a}\ne 0\).
From \((B)\), let \(\dfrac{a-H}{a}=\dfrac{1}{r}\). Then the expression \((A)\) holds.
Therefore, for positive numbers \(a, b\) and \(H\),
‘\(a\ne b\) and \(H=\fbox{\(\;(\beta)\;\)}\)’ is \(\fbox{\(\;(\gamma)\;\)}\) for a real number \(r\) to exist such that expression \((A)\) holds.
In the proof above, what are appropriate for \((\alpha), (\beta)\) and \((\gamma)\) in this order? [1.5 points]
  1. \(\dfrac{H-b}{b}\), \(\dfrac{2ab}{a+b}\), necessary and sufficient
  2. \(\dfrac{H-b}{b}\), \(\dfrac{ab}{a+b}\), necessary and sufficient
  3. \(\dfrac{H-b}{b}\), \(\dfrac{2ab}{a+b}\), sufficient
  4. \(\dfrac{b-H}{b}\), \(\dfrac{2ab}{a+b}\), necessary
  5. \(\dfrac{b-H}{b}\), \(\dfrac{ab}{a+b}\), sufficient
For all positive numbers \(n\), the polynomial function \(f_n(x)\) has the following properties.
  1. \(f_1(x)=x^2\)
  2. \(f_{n+1}(x)=f_n(x)+f_n'(x)\)
What is the constant term of \(f_{25}(x)\)? [1.5 points]
  1. \(548\)
  2. \(550\)
  3. \(552\)
  4. \(554\)
  5. \(556\)
On the \(xy\)-plane, there are two points \(\mathrm{O}(0,0)\) and \(\mathrm{A}(2,0)\), and a point \(\mathrm{P}(t,2)\) moving on the line \(y=2\). Let \(\mathrm{Q}\) be the point where line \(\mathrm{AP}\) meets the line \(y=\dfrac{1}{2}x\). Let \(t_1\) be the value of \(t\) for which the area of \(\triangle\mathrm{QOA}\) divided by the area of \(\triangle\mathrm{POA}\) is equal to \(\dfrac{1}{3}\),
let \(t_2\) be the value of \(t\) for which it is equal to \(\dfrac{1}{2}\), \(\cdots\), let \(t_n\) be the value of \(t\) for which it is equal to \(\dfrac{n}{n+2}\). What is the value of \(\displaystyle\lim_{n\to\,\infty}t_n\)? [2 points]
  1. \(0\)
  2. \(1\)
  3. \(2\)
  4. \(3\)
  5. \(4\)
What is the maximum value of the function \(f(x)=\log_9 (5-x) + \log_3 (x+4)\)? [1.5 points]
  1. \(\dfrac{7}{2}\)
  2. \(4\)
  3. \(\dfrac{2}{5}+\log_3 4\)
  4. \(\dfrac{3}{2}+\log_3 2\)
  5. \(4+\log_3 6\)

Mathematics·Studies (I)

Nat. Sciences
Three points \(\mathrm{P, Q}\) and \(\mathrm{R}\) on the \(xy\)-plane satisfy the following.
  1. Points \(\mathrm{P}\) and \(\mathrm{Q}\) are symmetric about the line \(y=x\).
  2. \(\overrightarrow{\mathrm{OP}}+ \overrightarrow{\mathrm{OQ}} =\overrightarrow{\mathrm{OR}}\) (※ \(\mathrm{O}\) is the origin)
Suppose point \(\mathrm{P}\) moves on a unit circle with the origin as its center. What kind of shape does the point \(\mathrm{R}\) move on? [2 points]
  1. A point
  2. An ellipse
  3. A line segment
  4. A hyperbola
  5. A parallelogram
Let \(x\) be the amount of labor input and \(y\) be the amount of capital input for some industry. Then, the output \(z\) of the industry is known to be as follows.
\(z=2x^\alpha y^{1-\alpha}\) (\(\alpha\) is a constant with \(0<\alpha<1\))
According to some data, the amount of labor input and capital input in the year \(1993\) was \(4\) times and \(2\) times that of the year \(1980\), respectively, and the industry output in the year \(1993\) was \(2.5\) times that of the year \(1980\). From this data, what is the value of the constant \(\alpha\) calculated to the hundredth?
(※ \(\log_{10}2=0.30\)) [2 points]
  1. \(0.50\)
  2. \(0.33\)
  3. \(0.25\)
  4. \(0.20\)
  5. \(0.10\)
Suppose we want to buy three rectangular iron plates, and make a gas boiler in the shape of a solid cyllinder as shown to the right, using two plates for the top and bottom faces, and the other one for the body. We can buy each iron plate with any horizontal and vertical length as we desire, and the price of an iron plate is \(10,\!000\) won per \(1\text{m}^2\). What is the minimum cost needed to buy the iron plates to make a gas boiler with a volume of \(64\text{m}^3\)? [2 points]
  1. \(1,\!110,\!000\) won
  2. \(1,\!040,\!000\) won
  3. \(1,\!000,\!000\) won
  4. \(960,\!000\) won
  5. \(900,\!000\) won